Seeking a Language in Mathematics 1523-1571

Bruce Marsden Scope

Cuthbert Tunstall, later Bishop of London and then Durham, published the first book on mathematics conceived and printed in England in 1523; it was a commercial arithmetic written in Latin. By the time of Thomas Digges's publication of his book on geometry in 1571, the use of English in mathematical publications and the practical arts' had become established, but not entirely to the exclusion of Latin. There is a parallel, which may be aetiological, between the growth of the use of vernacular languages and the striking surge of mathematics in science in western Europe culminating during the seventeenth century in the works of Descartes, Galilei, Huygens and, particularly, Newton.

The period under consideration is the beginning of what has become known as the Scientific Revolution,[1] which is usually agreed to span about 1550-1700 and during which mathematics became the distinguishing discipline. Just as significant, however, is the bearing of mathematics on the evolution of technology with regard to the much later Industrial Revolution, from about 1750. Interaction between science and technology is another tale to tell, and there is an important overlap in the making of scientific instruments. In the first decades of the Scientific Revolution the most notable instruments were to do with navigation, which connects to cosmology and astronomy in one intellectual direction, and to the surveying of land and buildings in another. These bonds will become evident in the works of Robert Record (c.1510-58), John Dee (1527-1608), and Leonard c.1510-57) and Thomas (c.1543-95) Digges, the main authors considered in this essay. The languages (verbal and symbolic) for basic mathematics (arithmetic, geometry and algebra) were formed in principle, and the means whereby the languages were to be developed were broadly indicated.

In addition to the growth in the use of English for mathematical works, other related topics to be considered include: the value of such publications for artisans (Shakespeare's rude mechanicals'), and in education more widely; contributions to the English language from mathematics; developments within the study of mathematics of the use of mathematical symbols and notation; and the growth of knowledge and understanding in mathematics in theory and practice (formerly, and improperly, pure and applied mathematics).

Verbal and symbolic language

The word 'language' is intended to mean one of two forms of communication. The first is the spoken and written language used in expressing the mathematical terms and operations; the second, the symbolic form of those terms and operations. This second treats notation, formulae, calculations and suchlike and is — in so far as these are held in common — understood internationally, whereas the first is understood only by those who are familiar with the spoken or written language of address. As will be seen, the verbal symbolic languages often worked in harness, with the symbolic form growing extending its influence gradually throughout the period. The most important single for in this growth is the development of the use of mathematical equations, which to be transformed by the introduction of the sign for 'equals' — this by Recorde in 1557 but it was not until the next century that the effects of his innovation were to be found in the literature.

Mathematics in English

It is a truism, but an important one none the less, to say that English was preserved and nurtured in the speech of the lower classes during the Middle Ages. By a process of linguistic osmosis in England English was becoming the main language of communication, ousting Anglo-Norman French in court and government circles, and competing with Latin in intellectual and theological affairs.

Following the introduction of movable-type printing in the latter half of the fifteen century, the movement gained considerably in momentum. Leonard Digges in 1556 wrote of the 'arte of numbring [which] hath been ...hyd and as it were locked up in strange tongues'[2] The strange tongues are primarily Latin, followed by French, Italian and German. But another tongue is the symbolic language of mathematics, a language frequently associated with the magic and sorcery.[3] In producing the early, and original, printed works on mathematical topics the verbal and symbolic forms were developed the same time in English once the groundwork had been established. This was done initially by direct and indirect translations, and also by transforming largely unwritten information used mainly in trade, commerce, and management of estates.

Before 1551 there was little innovation in the language of mathematics, as authors were mainly concerned with bringing into English concepts familiar though not written in English, and in translation from Latin and continental vernaculars at an elementary level on subjects which had a spoken form in English. With everyday arithmetic there was no problem putting into English the basic procedures, but with geometry and algebra it was altogether different. This is seen most clearly in the work of Recorde in 1551 when he attempted to anglicize the verbal language of geometry, which had already well established Latin and Greek forms of expression.

Debris from the battles for ascendency litter the pages of printed books with experimental words or constructions; some remained to provide synonyms with origins from earlier forms of English, the pre-Conquest invaders, and from French and Latin; some did not survive; others thrived.[4] Only the fittest forms of expression survived: those that were suited to their environment, those which could adapt or mutate, and those which offered a basis for future development. Comparable forces were at work on the symbolic language in mathematics. Seldom however have the lines been so clearly drawn as in the battle for the use of emerging new English in mathematics.

Imagination and mathematics

Verbal language, including the written form, is a means of recording and communicating; but is also an analogue for thinking which becomes condensed into the mental form of words before they are expressed externally. The symbolic language of mathematics is very similar for mathematicians, and but poorly appreciated by non-mathematical minds. History is written in words, not numbers.

In science, mathematics and technology there are general and specialist cultures from which each person draws, but to which not all contribute.[5] Naturally enough the more significant contributors attract the attention of commentators, leaving the foot-soldiers as footnotes.

As in many fields of human endeavour, there are heroes in mathematics; and there are cooperative activities: both are necessary. Who is to say which is more important — innovation or developmental advancement? Hero or developer? Heroes, by and large, tend to be innovators: Archimedes, Newton, Euler, Einstein. They are the generals; little, however, is heard of the developers, the other ranks.

In the period considered in this essay, the main authors are concerned with reconstituting the mathematical knowledge which had been known previously in Greek, Arab and medieval times; with translating it into English for a new readership; with developing certain features for immediate use, such as land surveying and navigation; and with achieving clarity of thought and of expression for communication to those who are not yet skilled in mathematical matters, or who wish or need to develop higher skills. In Recorde's books we can be observe the human mind grappling with concepts, seeking clearer modes of expression and paving the way for contemporaries and future toilers in the field. He was not always successful, and this is informative in examining his contributions.

The facility to think and outwardly express work in the same language favours innovation and development in the work and in the language. This is because the nature of the creative mind includes such apparently wasteful activities as 'dreaming' or mulling over matters in a non-linear way, conceiving situations of varying practicality, speculating, or simply walking round a problem to view it from different angles.[6] Likewise the process of putting into written form these abstract thoughts is made easier when the language of address is the same as that in which the products of thought are crystallized.

One debatable edge that emerging English was developing over Latin was a relative freedom from highly pre-structured thought patterns and the firmly established range of the hinterland of meanings associated with words and word groups. A word in English grown as it were organically could relate far more closely with human experience; whereas with Latin, when set apart from Roman culture, there was always a distance and authority problem in thinking creatively. This became especially evident as education filtered 'downwards' in society — and this was occurring in the time of the Reformation in England. For reasons such as these there were various attempts to limit Latin influences in the emerging English; equally there was a movement to embrace 'inkhorn' terms.[7]

This battle led to an enrichment of the English language which has proved flexible enough to embrace words of different origins but with shaded meanings, increasing the range from which the choice for a particular meaning any be drawn. As in the verbal language of mathematics, so in the symbolic.

Mathematics in society

Practical mathematics, as in surveying, navigation or accountancy, are integral with affairs of any time; these times, although politically turbulent, were of relative stabilty in the London region. This was the centre for trade, industry and government, and was the most populous city in the country, with about 40,000 to 70,000 people of a population growing from about two and a half million to four million during the sixteenth century. London was also, overwhelmingly, the centre of the book publishing industry.

In a commercial environment mathematics was used for: counting, measuring (including areas, volumes, weighing), converting currencies, calculating profit and loss and dividing shares between investors. Furthermore, levies and taxation on trade and assets required procedures involving mathematics. As trade increased, education plays, a growing part in society, particularly for the young and the ambitious.[8] Education needed teachers and books, and very often it was the teachers who wrote the books — Robert Recorde, for example. Publishers and printers of books served the growing markets, sup plementing the far more numerous and profitable publications in religious matters and literature.

Despite the upheavals connected with the Church and state during this period, particularly during the 1530s, ecclesiastical functions required servicing. The Church calendar had to be maintained annually, requiring astronomical knowledge, observations, calculation, and publication (its calendar was much in need of reform). Among the earliest products of the printing presses were calendars, almanacs and prognostications.[9] These made use of material gathered for determining the dates of religious festivals, together with much miscellaneous information such as astrological and weather predictions, useful to farmers and coastal pilots amongst others.

As the international shipping trade increased, more sophisticated methods for navigation became necessary. These involved geometry and astronomy, and necessitated instruments to facilitate the observations, as well as to produce tables recording essential information concerning the position of the sun, moon, planets and stars. Books on the geometry of the sphere and on astronomy provided information for navigators as well as for religious purposes.

The dissolution of the monasteries and the redistribution of the wealth and incomes of the Church were to have dramatic effects throughout society, not least in that full information about the lands and properties was required urgently. How to measure land and buildings; how to evaluate assets in terms of money; how to divide the acquired assets; how to organize taxation and revenues. This can be readily perceived in the printing history of the time; the little book by Rychard Benese (1537) is the first to treat measurements for buildings, land and building materials, though John Fitzherbert had included land surveying in one of his books published in 1523.

Document types

Possible manuscript sources for early authors in English on mathematical subjects 1523-71 fall into distinct groups, some more well-filled than others. The following illustrates some of the complexity of potential sources for mathematics, and also for science and technology:

ancient texts in direct translation into English from Greek and Latin (and also into other vernaculars);
ancient texts by way of Arabic into English from Greek and Latin (and also into other vernaculars);
ancient texts by way of Arabic into Latin; 
ancient texts into Latin in post-Roman times;
medieval texts from Latin into English (and also into other vernaculars);
medieval texts from French (and other vernaculars) into English;
medieval texts in Latin;
medieval texts in English;
medieval texts in other vernaculars.

Sources for Arabic texts would include eastern Mediterranean and Hindu, mainly; in addition to the ancient Greek and Roman cultures.

Some of the texts remained in manuscript, others were to be published after the introduction of movable type in about 1460.

Possible printed sources for early authors in English on mathematical subjects 1522-71 would have been:

original works in English;
original works in other vernaculars translated into English; 
original works in other vernaculars;
original works in Latin published in England and elsewhere; 
works in Latin and Greek translated into English; 
works in Latin and Greek translated into other vernaculars; 
works in one vernacular translated into another.

Other publications to be noted here are not considered sufficiently significant here for authors working in the English language: works in English translated into Latin; works English translated into other vernaculars; works in other vernaculars translated into Latin.

For the purpose of charting the development of verbal and symbolic languages in mathematics, the main works to be considered fall into the section denoted 'original works in English'. By 'original' is meant 'conceived and written in English for the English reader'.

If plagiarism is taking from one book without attribution, and research taking from more than one (whether or not attributed), then the same criteria also apply to translations. Attributions and references to other works in the period discussed are not all that common, though if a book is a direct translation from a single source this is usually acknowledged. In discerning significant developments in the contents of books on mathematical subjects there are those which are innovative to the subject, and those s r new ways of presentation or interpretation, mainly in printing and the use of language.

Works of the main authors

Although several authors are discussed here, the four most notable are Robert Recorde, Leonard and Thomas Digges, and John Dee. Arguably the content of the work initiated in English by these authors in mathematics, astronomy, navigation and particularly in technology, has had a greater effect on present-day life than any other aspect of the printed word in the time of the Reformation. But the value of their work as judged at the time should not be over-estimated.

In the small city that London then was the informal meetings of Robert Recorde, Leonard and Thomas Digge, and John Dee with others, made a network which has been largely ignored subsequently, but the importance of which is now recognized.[10]

In the space of some fourteen years, 1543-57, Robert Recorde published four works on mathematics, and one on medicine.[11] The mathematical works became standard educational textbooks of the time continuing, with varying degrees of durability, into the seventeenth century.[12] In 1551 he campaigned for the use of certain kinds of words mathematics, but in 1557 recanted, though not wholeheartedly. Some obsolete forms expression, like Darwinian dead-ends, throw light on the survivors.

Recorde's publications on mathematical subjects all have evocative titles, befitting an author with literary aspirations: The Grounde of Artes (arithmetic), 1543; The Pathway to Knowledge (geometry), 1551; The Castle of Knowledge (astronomy), 1556; and The Whetstone of Witte (arithmetic and algebra), 1557.

Leonard Digges, followed by his son Thomas, produced standard works on land ant: building surveying, Copernican astronomy and practical mathematics for the military. Thomas Digges (1571), importantly, ratified democratic cooperation with other languages as the way forward for English in mathematics. During his lifetime, Leonard Digges published two books: A Generall Prognostigation, 1553; and A Boke named Tectonicon, 1556. A third, A Geometrical Practise named Pantometria..., edited and enlarged by his son Thomas, was published posthumously in 1571, with a second edition in 1591.

John Dee was navigation advisor to the Muscovy Company (and was consulted by Frobisher and Davis). He offered a philosophical framework for the growing scientific knowledge of the time in the Mathematicall Preface to the English translation by Henry Billingsley of Euclid's Elements of Geometrie (1570).

Not only was 1543 the date of the publication of Recorde's first book (on arithmetic) but also — far more famously — the date of publication of the heliocentric hypothesis in De Revolutionibus Orbium Coelestium by Nicholas Copernicus.[13] Recognition of the significance of this has led commentators to proclaim the opening of the 'modern era' in science to be 1543. If that year can be claimed for science then, arguably, the same can be claimed for British technology on behalf of Robert Recorde.

Printed works 1481-1523

William Caxton: Within about five years of setting up his printing press using movable in Westminster, William Caxton published the earliest printed encyclopedia in the Myrrour of the Worlde, in 1481. As with so much else at this time it was a translation. Compiled originally in about 1245 in Latin and translated into French in as Image de Monde, it was considered to be suitable still in Caxton's time. This complacency was soon to change, as one of the effects of printing was to make readers are of the quality and reliability of the information contained in a book; and infelicities of language in translation quickened the search for suitable printed forms in which was developing rapidly, not just in words and phrases, but also in aspects of grammar, including sounds and rhythms. The introduction of the printing hastened the need as well as facilitated the process.

The Myrrour of the Worlde contained the earliest printed reference of some length to anything mathematical in English, with a few words on arsmetrique (number) and on geometrye (measure). In each of these sections is a statement as characteristic of the Ages as of the Age of Enlightenment,[14] and illustrates that the revival of Plato's philosophy in early humanist times had earlier soundings in the Scholastic studies of Plato. Thus, arsmetrique: 'Who that knewe wel the science of arsmetrique he myght see thordynance of alle thinges. By ordynance was the world made and created, and by ordynance of the Soverayn it shal be deffeted.[15] And geometrye: 'Who wel understode geometrie, he myght mesure in alle maistryes; ffor by mesure was the world made, and all thinges hye, lowe and deep.'[16]

Although comprehensible as English, one can sense the original language even in these extracts; in addition to English, most clearly are Latin. French and Germanic languages. With the addition, directly, of Greek, mainly through the influence of humanist studies, these were to form the basis of English in mathematics, science and technology.

Ephemera: In the early years of the sixteenth century popular almanacs, calendars, and prognostications were sold inexpensively.[17] The kalendayr of the shyppars[18] was the most widely published in English from 1503 to the middle of the seventeenth century and contained the movable feasts of the Church and tables for finding the Sunday letter. It also contained astrological information, details showing the entry of the sun into the zodiac, .+ to read the hour from the stars, and much else. Almanacs contained useful information about the weather,[19] eclipses, the best days for blood-letting and so on.

The content and language are revealing of the work of the translators in scientific literature, and in the first two decades of the sixteenth century that meant the calendars, almanacs and prognostications. There was a tendency to translate directly word for word, retaining the word order; where changes were necessary, syntax was not yet adequate and consistent; only a superficial knowledge was held concerning astronomy (which was the main 'scientific' content); and terminology in English was unsatisfactory. Comparison of Copland's 1508 version of the kalendayr of the shippayrs with Digges' Pantometria (1571) shows areas where development took place.

After about 1540 almanacs and calendars came to be published as one, while prognostications (usually on single sheets) were forbidden by statute (1541 and 1549) to issue false, politically unsettling, or socially disturbing prophesies; it was a nervous time, and the printed word carried considerable weight in the common mind.[20] Astrology and astronomy were hardly separable in the early years of the sixteenth century. Astronomy was beginning to become disentangled from myth and magic, but they were never far from each other even, after the publication in 1543 of Copernicus, De Revolutionibus.

Cuthbert Tunstall (1522): Of the many books in Latin on mathematical subject published abroad around 1500, it is noticeable that the most famous English author had been dead for more than a century and a half. This was the Merton scholar Thomas Bradwardine, renowned in his day but by 1500 outdated. Nevertheless, he was held such esteem on the Continent that in 1496 and 1503 his Geometria Speculativa and Arithmetica Speculativa respectively were published in Paris. Thomas Linacre trans. De Sphaera by Proclus (c.450 AD) from Greek into Latin; printed first in Venice reprinted in London by Richard Pynson in 1510, it was translated into English in 1550.

Cuthbert Tunstall (1474-1559) travelled in Italy as Henry VIII's representative. Concerned about being cheated in transactions with money-changers, he made notes from all he read on the subject and decided to bring them together in a Latin treatise De arte supputandi, which was published in London by Caxton's successor Richard Pynson in 1522, the year Tunstall was elevated to the Bishopric of London. Tunstall dedicated the book to his friend and travelling companion, Thomas More, providing an interest account of the genesis of the book in a prefixed dedicatory letter.[21]

The mathematical contents of the book are unremarkable, showing convention medieval methods of computation and providing examples, together with an appendix tables for estimating the value of various currencies. Tunstall explains the method working of subtraction from right to left (seven minus four) instead of from left to right — (four from seven), which the Arabs practised. This he attributed to a presumed Englishman of whom nothing is now known, one John Garth. This seemingly minor matter is of considerable importance in regularizing procedures for mathematical equations, though Tunstall himself wrote out procedures of computation in full, as was the custom. Where he did use numerals they were Hindu-arabic including zero, and not roman.

The book was never translated into English but went through several continental editions. Erasmus praised the man and the book,[22] and the first printed version of the Greek text of Euclid's Elements was dedicated to Tunstall by the publisher Simon Grynaeus in 1533. Because Tunstall wrote in Latin and was published in Paris and Strasburg, his mathematical renown on the Continent far exceeded that at home. Becoming Bishop of London in 1522, and later Bishop of Durham, Tunstall seems never to have returned to mathematics.

Tunstall moved in governmental and Church circles, using Latin extensively, so to write his mathematical book in the same language was natural to him, especially bearing in mind that his sources were Latin as well as several vernaculars.[23] But the next books on mathematics in 1537 were in English; the tide of English was flowing. Between the date of publication of Tunstall's book and the next books dealing with mathematics, in 1537, there are many statements advocating with various degrees of enthusiasm adoption of the vernacular in printed works. The following is from Elyot's Castle of Health (1534):

If physicians be angry, that I have written a physicke in englysche, let them remember that the grekes wrate in greke, the Romains in Latine, Avicenna, and the other in Arabike, whiche were their own proper and maternall tongues.

The Arab he could not bring to mind would have been Averroës (ibn-Rushd). Only after could anyone in England dare add that St Jerome wrote the Vulgate Bible in his tongue of Latin, translating from several earlier and contemporary languages. Tyndale had done so in 1528, but from abroad, in The Obedience of a Christian Man.[24]

Printed works 1523-43

John Fitzherbert: In 1523 John Fitzherbert (d. 1531) published two short works, both of went through many editions to 1767, and with later academic issues.[25] One was on husbandry, and the other was The boke of surveyeng and improvmentes.[26]

The bulk of the contents is a handbook for an estate manager or steward on such matters as rights of tenants, marriage, inheritances, freemen and bondsmen, and includes extensive notes on exactly how to perform the rituals of homage. The book, writes Fitzherbert, is taken from 'an olde statute named Extenta manerii ... made soone after the barones war the whiche ended at the bataile of Euesham' (1265), and compiled to bring under control the properties of the defeated parties. Each page of Fitzherbert's book is headed with the title of his source, but it is clear that he incorporates things of his own, including social comment.

In the introductory promotional verse passage, the publisher Thomas Berthelet calls the classical authority of the farming books of ancient Rome: those by Caton, Columnella, Varro and Vergilius. Because Fitzherbert gives no rules or procedures for actal measurement, it can reasonably be assumed that the primitive methods found in, example, Columnella's De re rustica, are to be taken as read.[27] In the introduction proper, he offers the origin of the word 'surveyor':

The name of a surveyour is a frenche name/and is moche to say in Englysshe as an overseer. Than, it wolde be knewen/how a surveyour shulde overse or survey a towne or a lordshyppe/as and the cytie of London shulde by surveyed ...[28]

Although there are no technical illustrations nor any numerical mathematics, there is an interesting account of procedures for surveying. The surveyor:

must stande in the myddes of the flatte whan he shall butte truely, and He that shall vieu/but and bounde landes or tenements by Eest West Northe and Southe. It is necessarie that he have a Dyall with him/for els * the sonneshyne nat/he shall nat have perfyte knowledge/whiche is East West Northe and Southe.

'[B]ut [or butte] and bounde' is a technical term long out of use in which 'to but' has the same sense as 'to sight', or 'take aim' as in archery practice (the 'butts').[29] The 'bounde' is the extent of the land surveyed, or boundary. 'Dyall' here is clearly a magnetic compass.[30] Thus with a perch rod (usually sixteen and half feet long), a magnetic compass and something to draw on to a scale, the boundaries were delimited and the shape of the parcel of land defined from a central position in the field. Practical advice in published form in English on how to determine areas of plots of land is not found here, suggesting the work of the 'overseer' is simply just that; and that the term surveyor as now understood had not yet taken on its present meaning. The change may be ascribed to the next book on surveying, by Sir Rycharde Benese, in 1537.

Book Trade Regulations: That Fitzherbert was treating the management of private in his book of 1523 and Benese was concerned personally with an ecclesiastical 1537 is a testimony to the turbulence of the times. Since the first act in 1484 to regulate the book trade, the conditions under which foreigners might carry on the business of printing and selling books were gradually tightened. There seems to have been a genuine wish to promote English practitioners in this expanding business, but there was also the matter of heresy and threat to social stability which had to be rooted out. As Bishop of London and Wolsey's right-hand man from 1522 to 1530, Tunstall had been involved, applying increasingly onerous restrictions on booksellers. In 1524 and again in 1525 he instructed them not the handle Lutheran works, in Latin or English, published in English or abroad. In 1525 Wolsey staged a book-burning event, to be followed by many others.

Promulgation of the Act of Supremacy in 1534 spawned numerous consequences among which official sanction to print the Bible in English became feasible.[31] In August 1535 Cromwell was asked for support to print Coverdale's Bible in English in England and in July 1536 ecclesiastical injunctions were issued ordering that every church was have publicly accessible the Bible in English and in Latin . The man who asked for support from Cromwell was the printer James Nicholson (originally a Netherlander), who was was rewarded for his audacity by being permitted to print the Coverdale Bible, with such success that two more revised editions followed in 1537.[32] Nicholson was also the printer for the maner of measurynge of all maner of lande by Rycharde Benese, probably the same year.

While the availability of a printed Bible was significant in that the Scripture now became accessible to all literate people, just as significant — some would say more so was the collateral effect within English-speaking culture more generally.[33] Having the Bible in English stimulated literacy, and consequently increased the demand for books of many sorts in English; it endorsed the English publication of subject matter that might otherwise have remained in Latin or Greek; and it created demand for original works in English.

In 1529 an act prohibited new presses being set-up by aliens, and in 1534 an act restricted the retailing foreign books to natives or residents in England, where publication could be more easily controlled at source. Commentators have been broadly of the view that this put the book trade firmly in the hands of native booksellers,[34] but actually several foreign printers and publishers became resident in England. One who did so as tension increased in advance of the enactment was Recorde's printer, Reyner Wolfe, in 1533,[35] and another is thought to have been the printer of the anonymous arithmetic in 1537, John Herford (or Hertford or Harford).[36]

Rycharde Benese: The maner of measurynge of all maner of lande, by Rycharde Benese (fl.537-47), brings the subject of surveying closer to modem practice by incorporating considerably more on measurement. Benese, a canon of the Augustinian priory of Merton, near London, published it at the dissolution, in 1537. The work is closely on measurement of land, buildings, and quantity of materials, providing many tables for multiplying numbers to provide areas, as though multiplication tables were not in use. The short Preface is by Thomas Paynell, also a canon at Merton, and he refers to the 'divine Plato', and to the usefulness of the contents for carpenters, masons, and 'those artificers who do use Geometry: by whyche all all maner of ingens and craftye ordinaunces of warre and other appertaynyng unto theyr arte do depende, as hangyng roofes, and galaryes, walles, shippes, galles, brygges, mylles, canes, wheles.'

Using one of the characteristic biblical expressions associated with neo-Platonism, Paynell reports that God made the world 'by number, weyght and measure'. Probably for the first time in English, particular aspects of the medieval system of dividing geometry mentioned in a printed work.[37] This classification is found in works on Practica geometricae, first formalized by Hugh of St Victor in about 1130, and subsequently developed and widely disseminated in scholastic circles until well into the sixteenth century.[38]

Benese himself, in the main body of the book, provides accurate ways of measuring of irregularly shaped fields by setting out a rectangle within the area, leaving triangular parts left over; the rectangle he deals with by way of simple multiplication using the tables he provides, and the triangles by means of multiplying half the height by the base. For regular figures of more than four sides:

Measure rounde about all ye whole circuite of thys figure * take the one halfe of the nombre of perches of that measure for the length. Afterwarde ye shall measure from the mydle poynte, within the figure, to the utter syde of the circuite, and take the nombre of perches of that measure for the bredth...

Benese also offers medieval procedures for measuring the height of hills and the depth valleys by means of triangles. There are twelve pages of text, including many tables for calculating volumes of timber, stone and areas for glass, and one page for calculating area of rooms.

His work was obviously well received, for after the dissolution he was made Surveyor Works at Hampton Court and chaplain to Henry VIII.[39] It is likely that his 'career move' was enabled by the knowledge expressed in his little book, and this in turn suggests that the practices he describes were not in the common domain. Stewards to the landed gentry probably had some knowledge of some or all of these simple operations, though numeracy was less common than literacy. Those with access to the medieval Practica geometricae treatises would also have known of these procedures, but perhaps have had little or no use for the information.

Anon. (1537?): The book printed by John Herford received little or no attention in modern times until 1947, despite it being alluded to by Robert Recorde in his arithmetic of 1543.[40] An introduction for to lerne to recken with the pen ... is a compilation from two anonymous continental sources, one Dutch (1508) the other French (probably c.1530).[41] Herford is thought to have been of foreign origin, but this far from certain.[42]

The book is a straightforward arithmetic treating seven 'species' — numeration, addition, subtraction, multiplication, partition (division), progression, and reduction. Books on arithmetic whether in manuscript or printed usually dealt with between five and nine species, later reduced to four.

All questions and answers are written out in full, there are no operative signs and numbers are Hindu-arabic including zero, as usual. Much of the text is taken up with examples of the 'rules' (that is, simple procedures in present-day terms). Numerous examples relate to everyday matters such as finding out how long it will take to fill a cistern if a certain proportion is filled in a particular length of time; this one, and many others involve the 'regula aurea called ye golden rule'.[43] Towns and coinage used examples to do with trade and commerce are usually French and English, though Dutch occurs in such as 'negenmannekens crownes'. The flavour of many of these early books and the type of problem treated is illustrated in the following extract:

    The rule & questyon of the walles.
    A manne wyll make a wall 32 fote in lenghte, and 2 of thycknes, and the heyght 25 fote, and eche fote shal cost the makynge 2 fs [francs]. I demaunde how moch shall cost the makynge of all the wall.
    Answere. fore to know this rule, ye shall mulyplye the lengthe by the thyckenes in sayenge 2 tymes 32 ben 64 & then ye shal multyplye it by heyghte in sayenge 25 tymes ben 1600, and then multyplye by the pryce, that is to wytte by 2 shyllynges the which ben 3200 shyllynges, whereof ye shal make francs, therefore dyvyde them by 20 and they ben 160 francs. An so moch shal coste the makynge of the wall.[44]

The printing history of this book represents one of the few, and possibly the last, examples of a religious establishment being involved in printing at that time. Between 1535 and 1540 acts promoting the dissolution of the monastic houses brought about a redistribution of a substantial part of the nation's resources, legal and illegal. Salvage from the closure of Abbey of St Albans probably included the printing press. This was the most advanced technology of the time, and of considerable value. John Herford's patron seems to have been Richard Stevenage, Abbot of St Albans: his personal device is found in several of the seven books Herford printed between 1534 and 1539, when the abbey was handed over to Henry VIII. The business was taken over by Nicholas Bourman in Aldersgate, London who began printing in 1539 and produced the second (?) edition in the same year. Bourman is said to have been a relative of Stevenage, transferring the business and probably the press as well when the abbey could no longer provide a place of work, though what happened to Herford during until he is next mentioned in 1544 is not known.[45] Herford produced the third edition, also with the Aldersgate address, in 1546.

Hugh Oldcastle (1543): John Mellis published A Briefe Instruction and maner how to keepe bookes of Accompts in 1588, as a 'newly augmented' version of one originally by the schoolmaster Hugh Oldcastle in 1543, which seems no longer to exist. It deals with bookkeeping in the Italian manner 'of Dare & Habere, which in our language of English, is called Debitor and Creditor', and is the earliest book in English to deal with double entry bookkeeping. It is based on the arithmetic section of Luca Pacciolo's Summa de Geometria, Proportione et Proportionalita (1494).[46] This, in turn, is the first comprehensive work after the Liber Abaci (1202) of Leonardo of Pisa (Fibonacci).[47]

It is likely that the need for a book of this sort in English in 1543 arose out of the need to deal with the increased accounting work associated with the dissolution of the monasteries. Adding nothing new to Latin and Italian treatises, its significance lies in the bringing into English the subject matter treated earlier by Tunstall in Latin. In the same year Recorde published his Grounde of Artes on arithmetic, beginning the publication of original works on mathematics in English.

Cultural climate 1547-58

Between 1543 and 1550 there were no books published on mathematical topics in English; then in the 1550s there was a spate of new work. The major mathematical books of the 1550s, abstract and practical, were responses not only to needs of the times but were firmly embedded in affairs affecting the stability of the nation, as the contents of prefaces demonstrate.

All the major English mathematicians of the generation (Recorde, Leonard Digges, Dee) together with the vitally important connecting link (Cheke), were, in the reign of Mary, in prison at one time or another on serious charges. They were not singled-out by the authorities because they were mathematicians, or in Cheke's case an influential champion, but more than sheer coincidence was surely at work. Emancipation of the artisan and merchant classes developed alongside religious emancipation, with concomitant pressure on the ruling families and their supporters. Scientists in Protestant countries did not have to refer to the Church for their authority or for its sanction on their work. Yet the Inquisition was continuing to be powerful in Spain a mere fifty years after the death of Torquemada, and was increasingly vigorous in Italy, culminating in the famous trial of Galilei in 1616 for advocating the Copernican system of the universe.

Sir John Cheke

The importance of John Cheke (1514-57) in the field of mathematics is not for any mathematical contribution, but for his promotion of the study of mathematics and for connecting the esoteric (and lowly regarded) discipline of mathematics to the outside world, including the publishing fraternity and influential court circles. He placed a cultural value on mathematics that was hitherto lacking among socially powerful, perhaps excepting only Thomas Linacre, and he succeeded in achieving some credibility for certain mathethematical pursuits, such as in navigation (and therefore exploration), where advantages for wealthy entrepreneurs and organizations could be perceived. As a scholar of Greek culture, he was also interested in Greek science, and actively encouraged its study; he was probably unique for his time in this endeavour. Cheke also had the foresight to place high value on the use of English, and fostered its early growth.

In 1540 at Cambridge, if not before, Cheke would have met Recorde, who was then studying medicine there. John Dee took his B.A. at St. John's in 1545, leaving for Louvain after his M.A. in 1547. On his return in 1550, Cheke introduced him to the Warwick family, two sons of the earl having heard his famous lectures in Paris year. Among Cheke's other pupils were Roger Ascham (author of Toxophilus and The Scholemaster), and later tutors in mathematics at Court William Buckley and Adams. There is no mention of a Leonard Digges in the Cambridge record, Cheke's sister Mary married William Cecil (afterwards Lord Burghley and chief secretary of state to Elizabeth).

Cheke had been appointed the first Regius Professor of Greek at St John Cambridge in 1540, at the age of twenty-six, and from 1544 to 1551 he was tutor to the future king, Edward VI, and to the Dudley family. He was examined as a witness in the trials of Bonner (1549) and Gardiner (1550), and was knighted in 1552.

When Northumberland was tried for treason in 1553, he blamed William Herbert, Duke of Pembroke for arranging the conspiracy. Whatever the division of culpability, Pembroke changed sides just in time, but left Cheke fully exposed in the position secretary to Lady Jane Grey. In July 1553 Cheke was imprisoned in the Tower for in the conspiracy, but was discharged in September 1554 with a licence that allowed him to travel abroad. However, he overstayed the period of validity of the licence, was in Brussels at the beginning of 1556, and brought back to London. Threatened with burning he capitulated, confessed and recanted publicly before the Queen and court in October 1556. He 'pined away with shame and regret' in September 1557. These are highly relevant to Recorde's parlous position at about the same time.

Recorde's Arithmetic (1543)

The Preface: Recorde alludes to an earlier work in English in the Preface to his Grounde of Artes (Arithmetic), 1543.[48] This can now be safely thought to be the anonymous work of 1537 printed by John Herford at St Albans. The publisher is named as R. Wolfe, an address at the 'sygne of the Brazon serpent' and S.T.C. equates R. Wolfe, Reyner Wolfe, Reynold(e) Wolfe and the anglicized Reginalde Wolfe; the D.N.B. gives Reyner and Reginalde as alternatives. However, records of 'denization' exist for a Reyner and a Reginalde; the first in 1533 and the second 1542.[49] (Interestingly, the date of first book published by Wolfe is 1542.[50])

One of Wolfe's earliest publications in his first year was a book in Greek by John Cheke (S.T.C. 14634) which required not only Greek alphabet letterpress but also skill the language to compose and process the production. Wolfe's ability clearly impressed Cheke, for when Recorde was ready to have his arithmetic printed it was to Wolfe that he went. Technical skill in handling Greek, and therefore probably arithmetic too, would certainly have been a consideration in the selection of a printer and publisher. Recorde had 'first saluted the Oxonian Muses' in 1525, went on the Cambridge and obtained his M.D. in 1545.[51]

The puzzle as to what John Herford might have been doing between his summons to Cromwell in 1539 and the next date of publication of a book by him in 1546 leads to the conjecture that he was working with Bourman, at least for part of the time, at an address close by St Paul's. Without affecting that guess another factor emerges in that the name of John Herford also appears in the imprint of a book published by Wolfe in 1544. Perhaps Herford was plying his trade as a journeyman printer during those years, or at least 'for hire' from time to time, when Bourman's business (which may have been Herford's under another name) was slack. Wolfe, Bourman and Herford are all thought to have been of Dutch origin, and it seems inevitable that they mixed socially and in business in the small printing quarter around St Paul's. It is also likely that Cheke learnt there anonymous arithmetic (1537) if he had not already been aware of it, and that this information was then passed on to Recorde. Equally, of course, Recorde may have seen it for himself, or been aware of it from another source.

Several early reference works, including the now outdated entry in the D.N.B. give 1542 (others give 1540) as the date of first publication of Recorde's arithmetic. These have arisen from John Bale's bibliographical researches. However in the introduction to the printed index to the notebooks, the point is made that Bales visited authors and printers' workshops to gather the most up-to-date information, and that in several cases date precedes by a year or two the actual date of publication.[52] It seems likely. that Bale (or another observer) saw Recorde's arithmetic in manuscript in Cambridge in 1540 or in the course of being printed in Wolfe's workshop in 1542, perhaps towards the end of that year. On the evidence of geographical proximity and of his name appearing on a work by Wolfe in 1544 it is possible that Herford himself was involved in some way with the production of Recorde's arithmetic. In addition to the earliest extant copy bearing the date 1543, there is internal evidence in support of that date. In the section on counters and counting (accounting), the first illustrated example of the of a counting board employs the number 1543, and this does not change in the later editions seen: not conclusive, but an indicative conceit.

Many of the 'problems' used in the anonymous book were later employed by Recorde, and this has led to the first use in English of some examples being attributed to Recorde. Several of the examples, however, go far back into antiquity, and a very well known one is found in an Egyptian manuscript possibly as early as 3400 BC[53] The extensive use of examples in western Europe was promoted by Leonardo of Pisa (Fibonacci) in his Liber Abaci (1202 revised 1228). He was also the first major mathematician in western Europe outside Arabic Spain (the main centre for Arab science up to the end of the eleventh century) to advocate the use of Hindu-arabic numerals including zero. Another highly influential writer, significantly from around the same time,[54] was the Englishman Sacrobosco, also known as John Holywood or Halifax. His De Arte Numerandi, written probably about 1240, was a popular text in Latin and was printed in least nine editions between 1488 and 1523.[55] Recorde's arithmetic and the anonymous work should be seen as bringing into English the robust but old publications on that subject deriving from medieval times.[56]

Mathematics was not part of the school curriculum at that time nor for long after,[57] and the instructions are given from first principles, written in a friendly tone, and directed vainly at education of the young: 'I have written in the forme of a Dialogue, because I judge that to be the easiest way of instruction, when the Scholar maye aske every doubt orderly, and the master may answere to his question plainely.' Recorde is adamant that Mathematical learning should proceed step by step, and laments the state of ignorance in mathematics in England, justifying his book: as arithmetic '...is the ground of all mennes affayres, so that without it no tale can be tolde, no communication without it can be longe contynued, no bargaynyng without it can be duely endyd, nor busynes that man hath justly completed.'

The Preface contains many of the sentiments repeated in subsequent works references to Plato, Aristotle and God abounding.[58] Recorde writes that if the book received he will go on to 'set forth such entroductions into Geometry & Cosmography as hytherto in English hath not been enterprysed...'. These were to be The Pathway (1551) and The Castle (1556), but there is no mention of the part two of arithmetic expanded second edition of the arithmetic, 1552), nor of the algebra of 1557. It therefore, that the projected series underwent change in the unfolding.

The main text: In the body of the work the usual processes are covered — numeration, addition, subtraction, multiplication, division, progression, the golden rule, double rule of proportion, and the rule of fellowship with time and without.[59] Recorde proceeds: '... arithmetike is a science or arte teachynge the maner and use of nombryng, & may be wrought dyversly with penne or counters, & and other ways...[60] These other ways, addition to the then familiar counting board, are the abacus and finger counting for he includes separate chapters. These latter two derive from Leonardo of Pisa, though Bede had advocated finger counting very much earlier.

Recorde accounts for his spelling of 'arithmetike': 'Bothe names ar corruptly Arsnetrike for Arithmetyke (as the Grekes call it) and Awgrym for Algorisme (as Arabyans sound it)...' But Recorde himself (or his printer, perhaps) is not wholly consistent and offers Arithmetike, Arythmetyke, arithmetyke, though the choice was extensive (as the O.E.D. entry shows).

Even at this late date, in the chapter headed 'Numeration', Recorde feels it necessary to distinguish between roman and Hindu-arabic number symbols: '684, that viC.lxxxiiii'. In doing so he demonstrates that roman numerals were themselves subject to change and development.

In 'Progression' Recorde provides the ancient 'rule of three': '... ye rule of proportions, whiche for his excellenciy is called the Goleden Rule: Whose use is by 3 nombers, knowen, to fynde out another unknowen, which you deserve to know...[61] He illustrates this:

Or verbally as 'If three is to eight, and sixteen is to x, what is x?'

The large Z used by Recorde to denote proportionality can be found in a publication in the United States as late as 1797, in a variant form, but by then it was well out of date. This sign for proportion will be shown to be a crucial element in Recorde's thinking concerning the introduction of the sign for equality (=), for which he is well-known, but the sign of which has not so far been satisfactorily adduced (but see pp. 201-3).

Because later editions were numerous, revisions and enlargements have usually been attributed to the two main editors after Recorde's death, John Dee and John Mellis. It was obviously in the publishers' interests to promote 'new' editions of a popular work, but examination of the 1552 edition, revised by Recorde himself, shows that all the major additions were included in 'The second Part of Arithmetike Touching Fractions, Brefely sette forth..' In this Recorde applies the same mathematical operations as in the first part to fractions.

William Salysburye (1550) and Anthony Ascham (1552)

The significance of these two small books, on the globe and on astronomy, lies not in the contents, which are elementary, but rather in the fact of their existence in English in printed form. Criticism of many of the early books on science and mathematics has been that they added nothing to the subject.[62] However this was a time of gathering up what gone before and presenting that information in an accessible manner; without such introductory works the next generation could not have moved forward — and the next generation was not far behind. The point has already been made that a major driving force in the Reformation was the youth of the day, and this is confirmed in the educational aspects of the publications on mathematics.

Salysburye's The Description of the Sphere or frame of the Worlde is a translation from the Latin by Thomas Linacre of De Sphaera by Diadochus Proclus.[63] The original Greek work was produced in about 450 AD and is a Ptolemaic view of the world. Linacre (c. 1460-1524) taught Greek to Erasmus and More, and was tutor to Prince Arthur before becoming physician to Henry VII and Henry VIII. He was an early champion of the New Learning, and, like Cheke later, did not exclude science.

In the introductory letter addressed to his cousin, Salusburye says that he had been asked to find a book on the subject in English, but had failed to do so in all the bookshops around St Paul's. He had been forced to chose one of three or four Latin versions to put into English, and selected the Proclus-Linacre edition, but not without misgivings:

But wolde God that he, whiche translated it into Laten, had taken so moche paine, as for his countre sake, as to englysshe the same also Englisshe was his natyve tonge. Greke and Laten as well knowen, where as Englysshe to me of late yeares, was wholy to lerne, the Latyn not tasted of, the Greke not once harde of, whom although even at this present I might rather and truelye with lesse reproche, denye to have any knowledge in it at all, than to professe the perfect phrase of any of theym three. Why than shall I attempt, for any mannes pleasure, to go aboute to translate a Scyence unknowen, out of a tonge unknowen, into a tonge no better knowen unto me.

His native tongue was Welsh. Once again the Welsh connection to science in Tudor times made.[64]

A lytel treatyse of Astronomye, by Anthony Ascham (published in 1552), was dedicated to the recently knighted Sir John Cheke, and presents a Ptolemaic interpretation of the universe. Ascham's seems to have been the first book in the mathematical field to provide a comprehensive index — although the entire publication is of only twenty-one folios.

Recorde's Geometry (1551)

The second new mathematical work by Recorde is The Pathway to Knowledge, containing only two of the four books promised.[65] It is dedicated to the youthful Edward not so much bycause it is the firste [book on geometry] that was ever sette forthe in Englishe...' but because he would be '... a wyse prynce to have a wise sort of subjects'. 'Nothing can be so grevous to a noble kyng, then that his realme should be other begerly, or ful of ignoraunce...' Dee's popular acclaim in Paris for his lectures on Euclid no prompted his older friend and colleague to publish The Pathway, though the book been promised in the Preface to his arithmetic in 1543. He also makes the first reference in modern times to Roger Bacon's 'perspective glasses'; by his remarks it seems that tests undertaken by Leonard Digges and John Dee had not yet taken place, but were to do so.[66] He also mentions the accusation of Roger Bacon being a 'necromancer'. writes that '... he never used that arte (by any coniecture I can fynde) but was in geometrie and other mathematical sciences so experte, that he could dooe by them such thynges as were wonderful in the syght of most people.[67]

His book will be useful, Recorde tellingly remarks, because there are 'a great nombre of gentlemen, especially about the courte, whiche understand not the latin tongue'.

Taking his cue from Thomas Paynell in the Preface to Benese's little book of 1539, Recorde extols the value of geometry to artisans, 'Carpenters, Karvers, and Masons doe. willingly acknowledge that they can worke nothing without reason of Geometric...' that geometry is of great practical worth to 'Merchauntes, shipmaking, navigation, compass, carpenters, etc, the carte and the plowe, tailers and shoomakers, weavers, milk, and all that is wrought by waight or by measure'.[68] So full of purpose is Recorde that he dedicates a page and a half of verse to the usefulness of geometry.

Recorde wryly comments on the social status of mathematical publications English:

I doubt not gentle reader, but as my argument is straunge and unacquainted with the vulgare tongue, so shall I of many men be straunglye talked of, and as straungly iudged. Some men will saye peradventure, I might have better employed my tyme in some pleasaunte historye, comprisinge matter of chivalrye.[69]

The contents of the work are a translation and rearrangement of the first four books of Euclid's Elements. As Proclus had done before him and Ramus was to do after him, Recorde separated the constructions ('things to be done') from the theorems ('things to be proved').[70] The most noticeable feature of the book is the introduction of words of 'English' (Anglo-Saxon) origin as new terms for more familiar Latin-based ones. Recorde's association with Sir John Cheke at Cambridge was probably productive in both directions: while Cheke had been a long-time enthusiast for the vernacular, his letter on seeking purity in the English language actually post-dates the work of Recorde in mathematics, and also of Turner in botany.[71]

In the chapter headed 'The definitions of the principles of Geometry' Recorde introduced many such terms: 'There is an other distinction of the names of triangles according to their sides, whiche other be all equal ... and that the Greekes doe call Isopleuron, and Latine men aequilaterum: and in englissh tweyleke.' '... all sharpe angles these the Greekes and Latine men do call scalena and in englisshe may be called novelekes, for they have no side equal.' '...called of the Grekes trapezia, of the Latin menmensulœ and of Arabitians, helmuariphe[?], they may be called in englisshe bordeformes.'

In addition to new words, Recorde also coupled words, linking a proposed or unfamiliar term with an established term or a variation of one. The following may not be complete, and as can be seen, most did not survive:[72]

    perpendicular or plumme line
    parallel or gemowe lines
    axelyne or axtre for centre line
    touch line for tangent
    cantle for half (or one of two unequal parts, used in connection with curved shapes)
    match corners for opposite angles formed by two intersecting lines
    cinkangle for pentagon
    siseangle for hexagon
    septangle for heptagon
    ground line for base of triangle
    tweylike or tweyleke for isosceles triangle
    threlike or threleke for equilateral triangle
    nonlike for scalene triangle (triangle had been in use since early medieval times)
    likejamme for parallelogram
    straight line for 'linea recta' instead of the more literal 'right line'
    a square quadrate for a square
    a long square for rectangle

Failure of most of the above terms to secure a place in the English language probably arises from two main handicaps. Their 'angular' sound is definitely old-fashioned for a time when the new language was responding more positively to subtler rhythms and less guttural utterances. As spoken words they fit more comfortably into Chaucer than Spencer. Perhaps more pertinently the family of words around, say, tweylike is highly restrictive when compared to the more expansive and geometrically better connected equilateral. Yet they survived in arithmetic in twain, twice, twelve, twenty, all from the Old Saxon root word 'two'. One of the keys to the process of enrichment of the English was the introduction of suffixes particular to English usage, allowing or encouraging adaptation of French and Latin terms already established in particular fields, such as geometry.[73]

Although the geometry in The Pathway is conventional, traditional for the time and familiar to later students capable of progressing beyond the pons asinorum,[74] one figure stands out in the Conclusion. This is the distinctive master mason's square; that with two arms at right angles but so tapered that the inner and the outer angles are both 90°.[75] This is a remarkably late example, if the interpretation of the drawing is correct, and and suggests a link between Recorde and practising Masonic geometers. But, true to their tradition, the Masons did not reveal its usage to Recorde, for he makes no specific mention of the square; he surely would have done so had he been aware of it. But the cannot have been drawn 'accidentally' and must have been intended. It is correctly located in the section treating the geometry of arches, and this makes it likely that he saw the master mason's square on site, probably at Cambridge.[76] The illustration in The Pathway does not seem to have been remarked on before now.

Recorde's Astronomy (1556)

Recorde's The Castle of Knowledge is his most famous book. It became the standard work on the subject, and held that place for over half a century.[77] Without elaboration Recorde tacitly accepts the Copernican system, referring to 'an infinite number of starres'. He might have been more fulsome had not Mary I been on the throne, and had he not been under threat of his life.

Recorde had been summoned to appear before the Privy Council on 20 July 1556, when he was bailed, but later he was confined in the King's Bench Prison, where he died in 1558.[78] This surely explains the supplicatory dedication to the 'Moste Mightie most Pvissant Princess Marye', and also remarks in the Preface concerning 'knowledge's exyle'. He is also referring to his actual or potential imprisonment in the following 'Althoughe I could not be permitted by disturbance of cruell fortune, to accomplish now my buyldyng as I had drawen the platte: yet in spite of fortune, this muche have I donne. Whiche is more then ever was done in this tonge before, as farre as I can heere.[79] The Preface becomes all the more poignant: read as a preface to a book on astronomy it is interesting and informative; read as a plea for a man's life it is enthralling. He is openly appealing, over the head of the Privy Council, directly to Mary.

There are two reasons offered for his imprisonment, the first 'for debt', and the second 'more likely due to some circumstance in connection with his work in Ireland'.[80] As no evidence has yet been produced for either, other possibilities should be considered. A more plausible cause for his imprisonment was his close association with Sir John Cheke and Leonard Digges, both attainted under Mary I and imprisoned in the Tower at about the time, or just before, Recorde was brought before the Privy Council. The devious actions of the illiterate Privy Councillor the Duke of Pembroke may be the connecting link; Pembroke was an ardent Protestant in a Catholic court, constantly in need of substantive proofs of his oft-questioned loyalty.[81]

While the Preface was surely written at this time, the main text was probably composed earlier. Recorde justifies the production of The Castle as being 'profitable' for navigation; when to sow grain, grass and planting; how to determine the dates for Easter, and for the 'right judgement of the Criticall daies that without it physicke is to be accompted utterly imperfect'. These 'daies' are those most favourable for blood-letting and the taking of medicines, important to Recorde, a physician. These practical reasons, we note, are exactly the same as for the kalendars and almanaces.

The Preface contains his usual references to Aristotle and Plato, rather more here than previously on God and Christ, and a personal version of a poem in the De Consolatione Philosophiae of Boethius:

[Man should]... rather looke upwarde to the heavens, as nature hath taught him, and not like a beaste go poringe on the grounde, and lyke a scathen swine runne rootinge in the earthe. Yea let him think (as Plato with divers other philosophers dyd trulye affirme) that for this intent were eies geven unto men, that they might with them beholde the heavens: whiche is the theatre of Goddes mightye power, and the chiefe spectakle of al his divine workes.[82]

Recorde's Castle was possibly the first book printed in England in English using roman typeface; that it was a book on science in the vernacular has been said to point to an important aspect of the intellectual revolution of the sixteenth century.[83] Whatever the background, the result is a modern-looking book easy on the eye. The publisher is named Reginalde Wolfe, the same name as he who stood surety for Recorde's bail in July most probably he had just anglicized it from Reynold. Wolfe did not publish anything else by Recorde, whose next and last book was published by the up and coming Kynstone (or, Kingston).

Recorde's Arithmetic and Algebra (1557)

By the time The Whetstone of Witte was published, Recorde was probably in prison. The dedicatory epistle, addressed to the 'Companie of Venturers into Moscovia', is dated 12 November 1557, and is more circumspect in tone than the Preface to The Castle.[84] The publisher may have been more prudent in allowing what was to be made public under their names, but Recorde's voice is still heard in the preliminaries:

I can not thinke it neadefull, to seke any protector, for this or any like worke. Sith every good man will offer hymself, to defende that, whereby his native countrie is benefited. Excepte at some tyme, by excitation of the furies, some naughtie natures doe practice their fraude, to berefte the realme of some singulare commoditie. But as I feare no soche, so at this tyme I seke no soche aide against them.

Whether this was bravado or whether he could not, or would not, involve colleagues in defence is not clear. Although three of his closest friends, Cheke, Dee and Leonard Digges, had all been imprisoned on treason charges and been released, they would not have been suitable 'protectors', had they even agreed to act on his behalf. Leonard Digges may even have been in prison at that time. Recorde seems to be claiming that his loyalty to the crown was epitomized in the benefit to the country of his mathematical work in English. Unfortunately his confident optimism was misplaced.

In the first part of the book devoted to expanding his earlier work, he uses the large Z for the sign of proportion. He also introduces about twenty-five symbols of mathematical notation, including one which, confusingly and needlessly, 'betokenth nomber absolute: as if it had no sign.' Although he tells his scholar pupil that he had received criticism for obscuring the 'olde Arte' by applying new names of native origin to established terms, he still uses 'cinkangle' and 'siseangle', and one or two new ones from his latest German sources, including for example, 'zenzizenzike roote' for 'fourth root'.[85]

In the second part, Recorde tells his pupil:

... now will I teache you that rule, that is the principall in Cossike woorkes: and for which all the other dooe serve.
    This rule is called the Rule of Algeber, after the name of the inventour, as some men thinke: or by a name of singular excellencie, as others judge. But his use it is rightly called the rule of equation: bicause that by equation of nombers it doeth disssolve doubtefull questions: And unfolde intricate ridles.[86]

Recorde goes on to introduce the = sign at the outset of the section on equations, giving the reason: '... to avoide the tedious repetition of these woordes: is equal to: I will as I doe often in woorke use, a paire of paralleles, or Gemowe lines of one length, thus:
__________
__________ , bicause noe 2 thynges, can be moare equalle.'

Note the pair of colons for quotation marks or italics which would be used today, and also the extended length of the sign itself. The telling phrase 'as I doe often in woorke use' indicates that he drew on his informal symbolic language for mathematics, and the significance of these few words should not be overlooked.

As the first line in the quotation above shows, Recorde uses the sign to replace ten words, as a form of shorthand; but in speaking the equation he would still have to have used the words he replaces on the written page. Lack of direct correlation between the spoken and written forms probably has much to do with the brevity of life of most of the mathematical symbols of notation which proliferated after about the end of the sixteenth century; and also why the abundance of other symbols essayed by Recorde in book failed to survive.[87] Just as in the verbal language for mathematics the words succeed are those that are specific enough to carve out a clear 'meaning', yet are flexible enough to allow adaptation, so for the symbolic language. The horizontal symmetry of an equation, being balanced on each side, the = sign forms a visual and mathematical fulcrum analogous to a Roman balance.

The basis of the ultimate success of the = sign lies in its simplicity and direct application to the established spoken terms. The origin of the sign may be just as simple. In the 'rule of three', the 'golden rule', Recorde used the large Z sign in his first arithmetic (1543) to indicate proportion, and to direct the user to multiply and divide in a particular sequence (provided that he/she knew the rules). Thus:

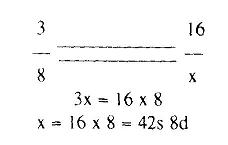
This is Recorde's own example, though he did not use 'x' for the unknown, leaving the space blank; nor did he use the sign for multiplication, but wrote the word out in full. As the resolution of the equation progresses on the page, the numbers are reduced from fractions to being on a single line; and, at the end of the procedure, a single numerical on expression — the answer required. The process of reduction at the same time makes the large Z redundant and too large or awkward to fit onto a single line; but a sign is still required for the condensed equation. By omitting the diagonal line and retaining the upper and lower parallel lines, the necessary compression is readily accomplished. What more natural as a shorthand for practical use, as Recorde confided in that neglected and revealing phrase, 'as I doe often in woorke use'.

The success of the = sign

The linear character of developmental equations was not fully established in Recorde's though the verbal form was linear because this is a characteristic of the spoken language, as it is of time. The development of mathematical operations and notation is paralleled by developments in the manner of speaking out the process.

One example of the non-linearity of many mathematical operations up to the six teenth century is the two-dimensional form of showing multiplication by means of a large X with numbers in the spaces between the four arms; another was for the numbers to be located at the end of each arm. In an analogous way to the large Z for proportion being transformed into the = sign, the large X became a small x within the line of the equation. This is first shown in the work of William Oughtred, published in 1631.[88] The = sign is only one of a group of basic symbols used in elementary mathematics, which include plus, minus, multiply, divide and equals; to which may be added signs for inequality and for proportion. Not only must they satisfy mathematical purposes but their use by printers when setting the type and in proofreading calls for consistency and visual clarity. Even at the end of the sixteenth century the difficulty in finding a printer capable of setting up a mathematical book was a cause for complaint.[89]

In 1659 the Swiss Johann Rahn employed the ÷ sign for division and this became the norm in Britain and the United States; but not in continental Europe where : was most commonly used, introduced by Gottfried Leibniz in 1684.[90] Although the + and — signs same into use at the end of the fifteenth century, there was very little agreement on the range of symbols until the nineteenth century, and even after that national differences persist.

There was nothing pre-destined about the ultimate success of Recorde's = sign: it could have been any other symbol to 'avoide the tedious repetition', for many were tried.[91] An abbreviated form such as aeq. could have survived, as could have the words including such as equals, aequales, aequantur, esgale, faciunt, ghelijk, or gleich. Recorde's remark about the inherent equality of the two parallel lines of the same length is apposite and conferred a relevance on the sign.

Like the = sign which pre-dates them, the signs for inequality — 'greater than' 'less than' — derive from the large Z sign for proportion employed by Recorde. The illustration in the book when the signs were made public in 1631 shows the graphical affinity with the = sign.[92] Hariot, following Recorde, used the long = sign, and he treated companion signs in likewise fashion, thus:

[93]

Recorde's contemporary John Dee seems to have been the first to use in print the : to denote proportion.[94] Later this was to become : between symbols or numbers on one side and :: between each side. The :: is familial with =, being simply the points at the end each of the pair of lines making the = sign.

Yet another relative is the sign in geometry for congruence, ≡ and neither did this sign find wide acceptance easily. Not until the middle of the nineteenth century was that particular version introduced, and not until the turn into the twentieth century did it gain wider acceptance.[95]

It was about sixty years before the introduction of the = sign was used again in print and the subsequent history is not straightforward. But as the 'family' of signs grew, the value of the simple = sign was perceived and absorbed successfully. It is probably true to say that by about 1700 the = sign was widely accepted and commonly (though not universally) in use.

The movement towards abstraction marked by increasing use of mathematical symbols, or generalization of principles, was evident before Recorde's time, and is indicative of a rapidly increasing intellectualization in mathematics culminating in the work of Newton and Leibniz in the next century.[96]

From the myriad of symbols used in elementary mathematics that emerged over several centuries, the ones that survived were those that have fitted their purpose in mathematical practice and in printing utility: no gauleiter was ordering affairs, and influences were diverse. There is an elegance, simplicity, and coherence between the fundamental operative signs which transcend their practicality. Choices were made, and it would not be too rash to include aesthetic considerations in the selection processes. Beauty, after all, can be a function as well as an adornment.[97]

Native and borrowed words (1557)

One of Cheke's last contributions to learning was the letter to Sir Thomas Hoby dated 16 July 1557 (if indeed it was penned at that late date).[98] The opening passage is well-known: 'I am of this opinion that our own tung shold be written cleane and pure, unmixed and unmangled with borowing of other tunges...' This appears to provide support to Recorde's efforts to employ words of Anglo-Saxon derivation in The Pathway (1551), but the less well-known following passage clarifies Cheke's intentions:

and if she want at ani tijm (as being unperfight she must) yet let her borow with such bashfulnes, that it mai appear, that if either the mould of our own tung could serve us to fascion a woord of our own, or if the old denisoned words could content and ease this neede, we would not boldly venture of unknowen wordes.

Put another way, words of English origin should be used where possible, but if it was necessary to import and adapt the appropriate words, then prudence should be observed. When Recorde reported in The Whetstone of Witte (1557) that there was criticism of his use of words of English origin in The Pathway (1551), it is very likely that Cheke had spoken with him about it in the interim.

Leonard Digges (1555, 1556)

Little is recorded of the early life of Leonard Digges and the date and circumstances of death are also mysterious.[99] Although he is reported as having attended Oxford, nothing is known of his college, the date, or degree, if any.[100] He is noted as working in an official capacity on surveying, navigation and gunnery in Calais, the last outpost of the king in France. He seems to have been born of minor landed gentry in east Kent, and thus well-placed to be required to be aware of the published work of Fitzherbert and of Benese. But he is next heard of under sentence of death for participating in Wyatt's rebellion, in which men of Kent were led to London early in 1554.

No known copies exist of Digges's first publication, A Generall Prognostication, in but the edition of 1555 is thought to be similar in content. What differs, however, is the Preface in which he refers to his 'late troubles' (imprisonment in the Tower) and the book as the result of his studies which 'might declare me thankfully mynded towarde your lordshippe [Sir Edward Fines, the dedicatee], emonge other honorable, to whome I myself, with all my endevore, the fruytes of my studye.[101] Unlike Recorde, Digges did have protection and was grateful for it, but maybe not for long, for he was attainted, apparently for a second time, on 9 December 1555 and presumably imprisoned again.[102] As there appears to be no record of his death, the likelihood is that this occurred before the accession of Elizabeth, otherwise he would surely have been released and published works which had been written: perhaps c.1557 is nearer the mark than the usual 1599.[103]

The subject matter of his Prognostications is in the tradition of earlier almanacs and prognostications, including information on weather lore, the ecclesiastical calendar, astrology, astronomical and moon tables, and practical sailing instructions including descriptions of instruments for navigation.[104] The quality of the information is more scientific than earlier examples of this type of publication, and its usefulness is confirmed the several editions down to 1635. The publisher was Thomas Gemini, a Flemish 'stranger' renowned for his engraving work and instrument-making. In 1552 he engraved a fine astrolabe with the arms of the Duke of Northumberland, Sir John Cheke and Edward VI, now in the Royal Belgian Observatory, Brussels.[105] Recorde and Dee were both connected to the Muscovy Company and to various expeditionary enterprises and probably Digges was also involved in some way, given his surveying and navigational expertise. When Leonard Digges was first imprisoned Dee took on the education of son Thomas, so they were clearly close.

It was Gemini's abilities in engraving which facilitated Digges' next book, in 1556, the last to be published in his lifetime, leaving several promised works unpublished, or perhaps un-written. Profusely illustrated with copperplate engravings, and in black letter, A Booke Named Tectonicon, ran to at least sixteen editions to 1637. In the Preface he writes:

... that the art of numbring hath been required (yea, chiefly those rules hyd and as it were locked up in strange tongues) they do profite, or have been furdered very little for the most pane: certe nothing at all, the Landemeater, Carpenter, Mason, wantyng the aforesayde:

But he cannot let himself claim absolute priority, for 'Other Bookes tofore put forth our Englishe tongue conteyned onely the bare measurying of Lande, Tymber, and Borde howe agreable in all places to the rules of Geomtery, let the learned idge.'

He goes on to assuage fear of technology:

Here (gentle Reader) thou shalt plainelye perceyve throwe diligent readynge, howe to measure truely and very spedely al manner of Lande, Timber, Stone, Pillers, Globes, Borde, Glasse, Pavements &c without trouble, not payned with many rules, or obscure termes...

In referring to projected works he mentions several times their completion being dependent on God sparing his life; for him this is not an idle phrase.

Digges certainly knew Benese's book, and he expands with suitable diagrams the same contents, employing the same method of measuring areas. Additionally he include an appendix on the use of the 'profitable staff' (cross-staff, or Jacob's staff). This is rudimentary instrument for setting-up similar triangles in order to measure heights and widths, and may be the same that Dee brought back from his visit to Louvain where he met the mapmakers Mercator and Finé. It was certainly well-known in continental Europe for many years previously.[106] Although he discusses the use of the mason's square the illustration is of a regular instrument, not tapered as the square represented it Recorde's Pathway.

Unusually for books of this time, and not occurring in any other mathematical work is his endorsement of teaching face to face. He concludes the Preface:

I would desyre where my grosse writynges seeme to be obscure, and I were presente the instructoure: for truely a lyvely voyce of a meane speculatour somwhat practised, furdereth tenfold more in my iudgement, then the finist writer. Farewell. Accept my good wyll, and Joke shortly (if God spare lyfe) for a profitable increase in these matters. Finis.

Whereas Dee and Recorde are known to have been renowned lecturers, Digges's standing is not registered.

John Dee (1570)

Of all the Tudor mathematicians Dee (1527-1608) is the most complex and consistently misunderstood, despite what should have been his rehabilitation in 1930.[107] Anyone with a deadline to meet must feel an affinity for the man as he rushes to conclude a section of most notable book: 'Tymes are perilouse: &c. And still the Printer awayting, for my pen staying'.[108] This illustrates the conversational aspect of many of the Tudor mathematical prefaces in English; of an author identifying with his reader directly.

Of Welsh descent, Dee went to St John's, Cambridge, in 1542 where he formed a life-long friendship with John Cheke. Dee read Hebrew, Greek and Latin, but also studied mathematics and no doubt met Recorde and others. On gaining his B.A. in 1547 he went to Louvain University where he met the major cosmographers of the day including ierard Mercator and Gemma Frisius.[109] He returned with mathematical instruments, particularly those to do with surveying and navigation, and in 1548 on taking his M.A. he left again for the Continent. After two more years at Louvain, in 1550 he gave his famous public lectures on Euclid's Elements in Paris. There he impressed and made friends with Peter Ramus (1515-72). These lectures were the first of their kind and made his international reputation. By 1570 and the time of the publication of The Mathematicall Preface to the translation by Billingsley of Euclid's Elements in 1570 his thoughts had matured, resulting in one of the most powerful and influential books in English that century.[110]

Probably the single most significant aspect of the Preface is the tree-diagram of scientific knowledge of the world under the general heading Sciences and Artes Mathematical, in which he distinguishes Principall and Deriuatiue. or theoretical and practical.[111] This is printed at the end of the Preface and includes words for many divisions of science which have not survived; words such as Anthropographie, Trochilike, Menadrie, Thaumaturgike, Zographie. Some names are conventional, but the definition given to architecture will illustrate that this paper is not the place to examine these words further.[112] Architecture is '...a Science garnished with many doctrines, and diverse Instructions: by whose iudgement all workes by other workmen finished, are iudged.'

He considered mathematics, and particularly geometry, to be the connecting link between them all, as well as uniting man to the cosmos. The Hermetic tradition in the neo-Platonism of the Renaissance as described by Ficino, Mirandola and Aggrippa was central to his thinking. Dee's complexity was such that an appraisal of his work would need to treat astronomy, mathematics, navigation, astrology, the occult and philosophy, in addition to ancient and contemporary authors in history, poetry and literature. The catalogue he compiled of his library illustrates the range of his interests as well as providing bibliographical information. Works are mainly in Greek, Latin, Italian, French or English, and the subjects are chiefly scientific, philosophical and of the occult, and not those of a literary humanist. The few theological works are not polemical standard texts of the Reformation or Counter-Reformation. One the notable scientific works is the twelfth-century translation from Arabic into Latin of Euclid's Elements by Adelard of Bath, and among other Arabic and medieval works are many by Jordanus, al-Farabi, Hazen, Robert Grosseteste and, particularly well represented, Roger Bacon.[113]

It would be an error to consider Dee's work as being a straight development from proto-sciences, such as alchemy, to the treatment of subject matter found in the Scientific Revolution of the next century. Herein is one of the fascinations that Dee's work produces: while he was clearly at the forefront of scientific knowledge and of technolgical advances in navigation, for example, he was also rooted in a fantastic mental world, difficult to comprehend today. This is probably the main reason why there is still no satisfactory all-round study of his work.

His library at Mortlake was probably the finest private library in Europe of the time numbering over three thousand books and a thousand manuscripts. It was formed as a result of the loss of many books and manuscripts at the dissolution and particularly it the time of Edward VI.[114] The sacking of the Oxford libraries in 1550 (that tempestuous year again) resulted in the destruction of books and manuscripts, especially those with mathematical diagrams, for they were accounted to be 'Popish, or diabolical, or both.' It is clear from this that, although it might have been a minority point of view, attitude, towards the New Learning in scientific matters were not always benevolent; life for practitioners could be fraught with danger. It was not a simple question of Protestant or Catholic, but more to do with fear of an apparently mysterious source of personal power — written-down knowledge, and compounded by diagrams and symbols as in mathematics, coded messages in diplomacy (maybe involving 'magic squares' using letters of the alphabet), and magic spells. Dee was active in all these spheres at various times of his life, and this facet of his work coloured his reputation then and thence into the present century.[116]

Dee's library was sacked by an angry mob in 1583, but he had at least made an inventory of its contents just before that catastrophe. He managed to salvage most of his books and manuscripts, but in his old age his daughter was forced to sell them one by one to pay for his upkeep.[117]

Dee's dedication to astrology enabled him to supplement his income from private readings; he was also employed at court as Royal Astrologer, drawing horoscopes for Mary, Elizabeth and courtiers. In this capacity he ran foul of Mary, or someone close to her, as he was tried for treason early in 1555 on the grounds of attempting her death. Most probably he drew her horoscope and possibly discussed the fatal features of it with Elizabeth, but he managed to talk his way out the trial at the Star Chamber. Even so, he was still imprisoned in the Tower under Bishop Bonner, who was instructed to examine him on religious matters, but found nothing untoward. Dee was released in August 1555, and at the beginning of 1556 felt himself to be in good enough standing with Mary that he petitioned 'for the recovery and preservation of ancient Writers and Monuments.'[118] His proposal was to salvage the manuscripts and books that remained from the dissolution of the monastic houses and sacking of the libraries, and to form a national collection. He failed to elicit support for this, and so began to expand his own collection with that end in view.

William Cuningham (1559)

Curiously, although Dee was certainly aware of the work of Gemma Frisius, having met in Louvain, he appears not to have brought back an essential element of surveying, or if he did, he kept very quiet about it: it does not figure in his own work or that of his close colleagues Recorde and Digges. This is the concept of triangulation. Although Dee always wary of making his work public, the book by Frisius was widely available in continental Europe.[119]

Triangulation is the system whereby each point is located by three lines of survey, fixing it in two dimensions, and remains the standard method today, though obviously considerably improved. With the length of one line known, all others follow without measurement by means of drawing to a prescribed scale using a pair of compasses. Or, with certain dimensions known, a check can be run on the accuracy of work in the field recording, in the case of rectilinear shapes, the diagonals. Thus difficulties of the terrain are avoided. All this Frisius treats in his book, and it is an unremarked-upon mystery that the topic was not mentioned in print in England until 1559. This was the subject for a part of The Cosmographical Glasse, by William Cuningham (1531-86).

In contrast to 'butting and bounding', triangulation enables the surveyor to chart great distances between 'station points', usually taken as church towers or other highly visible landmarks. It was this technique which enabled Christopher Saxton to produce, during 1573-79, the first detailed national atlas of any country.

Thomas Digges (1571)

In Prognostication, Leonard Digges referred to works completed but not yet published. One of these is A Geometrical Practise named Pantometria, 'lately finished' by his son omas and published in 1571.[120] Divided into four 'books', the first three are by Leonard, edited by Thomas, and the fourth by Thomas himself. The titles of the first three books are Longimetria, Planimetria and Stereometria, treating geomterical figures t one, two and three dimensions respectively.

At last here is the fusion of scholastic Practicae geometricae with actual practice. Until this book left the printing press, the published geometries described either medieval rough-and-ready methods from the agrimensorial tradition of ancient Rome (Benese, 1537), or were treatises based more or less on Euclid's Elements (Recorde, 1551). With the publication of this book those three threads of medieval geometry are brought together. Its importance cannot be overestimated in terms of signalling the virtually complete absorption of the three written classes of medieval geometry into English.[121] What now seems to have been lost to permanent records was the empirical 'constructive geometry' of the medieval masons, because Digges in his passage dealing with the builders' square does not mention or illustrate the tapered form of the instrument which Recorde depicted in The Pathway of 1551.

Apparently for the first time in a publication in English, the fraction 22/7 is used in connection with areas and circumferences of circles, in the section headed Planimetria.

The Preface to the first three books was written by Thomas, and he refers to people still living who had witnessed the use of 'perspective glasses' for seeing objects at a great distance, and for causing fire.[122]

The Preface to the fourth book, on Platonic solids, by Thomas Digges is of great interest in the matter of language:

I have retained the Latin or Greeke names of sundry lines and figures, as cords Pentagonall, lines Diagonall, Icosaedron, Dodecaedron, or such like, for as Romanes and other Latin writers, notwithstanding the copiouse and abundant eloquence of their toung, have not shamed to borrow of the Grecians these and many other termes of arte: so surely do I thinke it no reproache, either to the English toung, or any English writer, where fitte words fayle to borrow of them both.

This pragmatic approach, rather stronger than Cheke's statement on the topic, has been the rule for producing new words in science in English ever since. In retrospect it may appear obvious that this should be so, but Recorde's attempts to bring words of Angle Saxon origin into the language of mathematics should not be under-appreciated. If Recorde's proposals were revolutionary with respect to the established Latin form, Thomas Digges's was the middle path, which became the norm in a very English way.

Thomas Digges proceeds in the Preface to recognize the value of the use of algebra in harness with geometry, a significant insight fulfilled by Descartes nearly a century later:[123]

I have adioyned every of their diffinitions, and so proceded to Problems and Theorems with such methode, as howe obscure, or harde soever they appear at firste, through the rarenesse of the matter: I doubt not but by orderly reading the ingenious student, having any meane taste of cossical numbers, shall finde them playne and easie.

The difference between the two parts of the publication, by Leonard and Thomas respectively, is plain to see. Leonard's is descriptive and lacking notated mathematics, whereas Thomas's is considerably more quantitative. Surprisingly, Thomas did not adopt the = sign.

According to Leonard, his section was written in or before 1555. Thomas's can be assumed to have been written immediately prior to publication. In a note at the end of Thomas's section, clarifying his credit for only that part of the publication, he gives his age as twenty-five. This would put his date of birth at about 1546, and not at c.1543, which is the date given in many of the standard reference works.[124]

While the work of Thomas Digges is important in the fields of artillery, astronomy, and navigation, it is beyond the scope of this paper.[125] He achieved an international reputation particularly in astronomy, advocating the Copernican theory half a century before Galilei, and was highly praised by Tycho Brahe. His fame was made with his Alae seu scalae Mathematicae, which was written in Latin, and clearly with an eye to his reputation and the European market. However, in the 1591 edition of Pantometria, he proposes to use only English henceforth:

And although publishing the same my Treatize Martiall Pyrotechnie and Artillerie in the Latin toong, I should I knowe greatlye amplifie myne owne and the admiration of such rare Mathematicians as at this daye live in all Nations of Christendome, from whome I have for farre inferior Inventions Imprinted in my Treatize 'ntituled Alae seu scalae Mathematicae, already received no small applause. Yet if I publish the same at all, I doe constantly resolve to doe it onely in my Native Language: As well to make the benefite thereof more private to my Countrymen, as to make thereby other Nations affect as much our Language...

This was written shortly after the attempted invasion by Spain in 1588, so national security would have been a concern; the extract also illustrates that the sudden growth of in England in the realm of scientific matters had left the continentals less able to communicate with the English, except through another international language. First Latin continued as the scientific lingua franca, then, by the mid-seventeenth century, it was accompanied and then superseded by French. Not until well into the nineteenth century continental scientists consider it worthwhile to become familiar with English.

Primary printed sources

Any book, document, usage or occurrence, referred to as being the earliest example, is said to be so in the light of present knowledge and the limitations of the author.

A line over a letter indicating elision is omitted in favour of including the letter itself. 'U' is altered to 'v' where appropriate. Otherwise spellings are as in the original works.

• S.T.C.-A. W. Pollard and G. R. Redgrave, et al., A Short-title Catalogue of Books printed in England, Scotland, & Ireland and of English Books printed abroad, 1475-1640 (The Bibliographical Society, Oxford, 1926. Revised and enlarged 2nd edition, 3 vols., 1976-91).

• D.N.B.: Dictionary of National Biography.

• O.E.D.: Oxford English Dictionary.

• E.E.T.S.: Early English Text Society.

• William Caxton), Gautier de Metz, The Myrrour of the World (William Caxton, London, 1481 [S.T.C. 24762], 1490, 1527?), E.E.T.S., Extra Series no. I 10, London, 1913.

• Cuthbert Tunstall, De Arte Supputandi libri quattuor (Rychard Pynson, London, 1522 [S.T.C. 24319]; and Paris 1529, 1535, 1538; Strasburg 1543, 1544, 1548, 1551). Source, Charles Sturge, Cuthbert Tunstal, p. 75, though this is probably dated.

• John Fitzherbert, Here begynneth a ryght fruteful mater: and hath to name the boke of surveyeng and improvmentes (Rychard Pynson, London, 1523 [S.T.C. 11005], and 1526 [S.T.C], 1533, 1539, 1543, 1546, 1555, 1560, 1567, 1767). Source, British Library Catalogue. S.T.C. is slightly more cautious on some dates.

• Syr Rycharde Benese, This boke sheweth the maner of measurynge of all maner of lande, as well as woodlande, as of lande in the felde, and comptynge the true nombre of acres of the same (James Nicholson, London, 1537?, and 1540?, 1563, 1565?, 1651). Source, British Library Catalogue. S.T.C gives 1537?, 1550?, 1553?, 1562-3,1565.

• Anon. An introduction for to lerne to recken with the pen, or with the counters according to the true cast of Algorisme, in hole numbers or in broken newly corrected (John Herford, St Albans, 1537? [fragment only, British Library?]; 1539 [N Bourman. Bodleian Library only? STC 14118], 1546 [John Herford, London]. 1556, 1574, 1581, 1595, 1629.

• Hugh Oldcastle, A Briefe Instruction and maner how to keepe bookes of Accompts Newely augmented and set forth by John Mellis Scholemaister (John Windet, London 1588. [S.T.C. 18794]. Originally by Oldcastle, 1543; not now existing?)

• Robert Recorde, [arithmetic], The Groundes of Artes (R. Wolfe, London, 1543); additional part by Recorde 1552. 27 editions to 1640. Editions 'revised' by Dee from — 1575; 'revised' by Mellis from 1582.

  • [geometry], The Pathway to Knowledge (Reynold Wolfe, London. 1551), and 1574, 1602.
  • [astronomy], The Castle of Knowledge (Reginalde Wolfe, London. 1556), and 1596.
  • [arithmetic and algebra], The Whetstone of Witte (John Kynstone. London, 1557), and 1596.

• Leonard Digges, A Prognostication of right good effect (Thomas Gemini, London. 1555). That of 1553 not extant. 11 editions to 1605.

  • A Booke named Tectonicon (Thomas Gemini, London, 1556). 16 editions to 1637.
  • A Geometrical Practise named Pantometria... lately finished by Thomas Digges (Henrie Bynneman, London, 1571), and 1591.

• William Salusburye, The Description of the Sphere or Frame of the Worlde (no publisher in copy seen, St. Chantes Inne, in Holbourne, London 1550). S.T.C. gives R. Wyer, same date, different address.

• Anthony Ascham, A lytel treatyse of Astronomy (William Powel, London, 1552).

• William Cuningham, The Cosmographical Glasse (Ioan Daij. London, 1559).

• John Dee, [known as The Mathematicall Preface] Preface to The Elements of Geometric of the most auncient Philosopher Euclid of Megara. Faithfull (now first translated into the Englishe toung, by H. Billingsley... With a very fruitfull Praeface made by M.I. Dee... (John Day, London, 1570). Reprinted 1651 and 1661.

• Thomas Digges, A Geometrical Treatise named Pantomentria..., as Leonard Digges.

Notes

[1]	The term has been attributed to Herbert Butterfield in the 1948 lectures which became The Origins of Modern Science, 1949. Reported by M. B. Hall in The Scientific Revolution (Macmillan, London, 1970), p. 1.

[2]	Leonard Digges, A Boke Named Technonicon (Thomas Gemini, London, 1556), sig. A2.

[3]

Roger Bacon, The Opus Majus of Roger Bacon, (c. 1280), translated by R. B. Burke, London 1928 (based on corrected text of Bridge's edition of 1900), p. 261. Distinguishes between mathesis (short middle syllable, meaning knowledge) and 'nathesi (long middle syllable, meaning divination), showing the long-time association of idea of mathematics and magic. The first book on mechanics by an Englishman in English was by John Wilkins, later Bishop of Chester, published under the title Mathematical) Magick, or Wonders that may be performed by tlechanicall Geometry (London, 1648).

[4]	Albert C. Baugh and Thomas Cable, A History of the English Language Routledge & Kegan Paul, London, 3rd edition, 1978), Chapter 8.

[5]	For a wide-ranging discussion on this subject, see The Structure of Scientific Theories. Papers from the Symposium held at Urbana, Illinois, 1969 (F. Suppe ed., Chicago, 1974).

[6]	First-hand and qualitative accounts by mathematicians and physicists in Jacques Hadamard, The Psychology of Invention in the Mathematical Field (Princeton U.P., 1945. Dover reprint of enlarged 1949 edition, New York, 1954).

[7]	Brief notices in H. S. Bennett, English Books & Readers 1475-1557 (Cambridge U.P., 1952, 2nd edition, 1969), pp. 166-177, and A. C. Partridge, Tudor to Augustan English (Andre Deutsch, London, 1969), p. 46.

[8]

A.G. Dickens 'The English Reformation as a Youth Movement', in The English Reformation (Batsford, London, 1964, 2nd edition 1989), pp. 334-8. There seems to be some truth in this, but as the expectancy of life of anyone born in the sixteenth century was less than forty years, this fact can colour later opinions.

[9]	E.F. Bosanquet, English Printed Almanacs and Prognostications: A Bibliographical History to the year 1600 (The Bibliographical Society, Oxford, 1917).

[10]

Francis R. Johnson, Astronomical Thought in Renaissance Europe. A Study of the English Scientific Writings from 1500 to 1645 (Johns Hopkins Press, Baltimore, 1935), pp. 138-9. Although Johnson refers to 1560-83, Dee was cultivating contacts immediately after his return from Louvain and Paris in 1550.

[11]	There may have been another on mathematics, The Gateway to Knowledge, but this now seems lost.

[12]

The numerous editions of his most popular works are testimony to this. Sir Christopher Wren's copy of The Castle of Knowledge is in the Bodleian Library, shelfmark Savile K.5(3), noted in J. A. Bennett, The mathematical science of Christopher Wren (Cambridge U.P., 1982), p. 127. Wren was installed as Savilian professor of astronomy at Oxford in 1661.

[13]

The profound intellectual and practical impact of this work lies beyond the scope of this study, largely because it took decades to work out the physical consequences. Within our period there was little or no perceptible effect on the English language resulting from either the concept or the book of Copernicus.

[14]	As can be seen in the works of Leonhard Euler (1707-83); for example, in the Introduction to the Additamentum 1, Methodus Inveniendi Lineas Curvas Maximi Minimive Proprietate Guadentes... (Lausanne and Geneva, 1744).

[15]	Caxton, Myrrour, (E.E.T.S. Extra Series, CX), p. 37.

[16]	Ibid., p. 38.

[17]	Bennett, op. cit., pp. 116-20, and list, pp. 278 ff.

[18]	Translated from the French Le Compost et Kalendrier des Bergiers (Paris, 1493) with new translations and editions to 1656.

[19]	Although the O.E.D. currently repudiates any Arabic connection, a recent tative work maintains the Arabic origin of the word as meaning 'climate Seyyed Hossein Nast, Islamic Science (The World of Islam Festival PublisCompany Ltd., n.p., 1976), p. 96.

[20]

Bennett, op. cit., p. 119, reports that one prognostication made in Flanders ened Henry VIII with war and misfortune. L. Thorndike, A History of Mats. Experimental Science up to the Seventeenth Century (8 vols, New York, 19 V (1941) Ch. 11, describes another, known as the 'Conjunction of 1524', in it was predicted that there would be a return to the Flood because all the p1. were to be in Pisces in February of that year.

[21]

Translation with comments in Charles Sturge, Cuthbert Tunstal: churchman scholar, statesman, administrator (Longmans Green & Co, London, 1938). : 72-4. More and Tunstall first knew each other as students at Oxford Univer, the 1490s. Tunstall was almost certainly involved in the education of Margar Gigs, More's adopted daughter, for she is noted as being exceptionally talent, this field; perhaps being the first English woman to be noticeably so (to men eyes). On the day before his execution, More returned to her the 'algorithmiL stone' (whatever that may mean),which he had carried as a memento.

[22]	Sturge, Ibid., p. 76.

[23]	Ibid., p. 72, translation of the dedicatory letter from to More.

[24]	David Daniell, William Tyndale: A Biography (Yale U.P., London, 1994), p. 229.

[25]	Authorship ('master Fitzherbarde') has been disputed. It is between John and more famous brother, the lawyer Anthony; but the S.T.C. has settled on John.

[26]	For the book on husbandry see G. E. Fussell, The Old English Farming Books, from Fitzherbert to Tull 1523 to 1730 (Crosby Lockwood & Son, London, 1947).

[27]	O.A.W. Dilke, Mathematics and Measurement (British Museum Publications, London, 1987). Chapter 5 treats, concisely, mathematics for the architect and surveyor in ancient Rome.

[28]	John Fitzherbert, the boke of surveyeng and improvmentes, introduction, unpaged. The slashes in the original are to distinguish words in close-spaced black letter text.

[29]	O.E.D. gives 'butt' as 'to mark out limits in surveying', but this sense is not quite right. Note that * signifies a symbol used for '&', or what later became a comma, or to fill in a line of type.

[30]	O.E.D. gives 1653 as the earliest use of the word 'dial' in the sense of 'surveyor compass', although used here in 1523. The term (magnetic) 'compass' came into use by way of the mariners' arts — see Waters, The Art of Navigation, pp. 21-32.

[31]	For a short review of the regulations affecting the book trade see Bennett, op. cit., Ch. 3.

[32]	Dickens, op. cit., p. 153.

[33]	Elizabeth Eisenstein, The Printing Press as an Agent of Change (Cambridge U.P.. 1979 in 2 vols., 1980 in 1 ), Ch. 4, 'The scriptural tradition recast'.

[34]	Bennett, op. cit., p. 31.

[35]	Taylor, The Mathematical Practitioners of Tudor and Stuart England (Cambridge U.P, 1954), p. 313.

[36]	E.Gordon Duff, A Century of the English Book Trade (The London Bibliographical Society, London, 1905, reprint 1948), p. 70.

[37]	Altimetry, planimetry, and stereotomy are mentioned, but not longimetry and crassitude.

[38]	A good general reference is Elspeth Whitney, 'Crafts, Philosophy, and Liberal Arts in the Early Middle Ages, Transactions of the Amercian Philosophical Society, 90, I (1990), pp. 57-163.

[39]	Taylor, op. cit., p. 168.

[40]

Not mentioned by F. R. Johnson, Astronomical Thought, 1935, nor Taylor, The Maathematical Practitioners, 1954. See A. W. Richeson 'The First Arithmetic Printed in English', Isis 37 (1947), pp. 47-56. Richeson asserts 1537 without question-mark, as does M. B. Stillwell, An Awakening Interest in Science during the First Century of Printing (The Bibliographical Society of America, New York, 1970). Neither the S.T.C. nor the British Library Catalogue note this edition, which must therefore remain questionable.

[41]	Question of sources raised by A. W. Richeson (Ibid.) were traced by P. Bockstaele, Notes on the First Arithmetic Printed in Dutch and English', Isis 51 (1960) pp. 315-21.

[42]	Duff, op. cit., p. 70.

[43]	Or, 'the rule of three', discussed more fully in sections on Recorde's arithmetic and algebra.

[44]	Anon., An introduction for to lerne to recken with the pen ... (1546 edition), sig. i., iiii.

[45]	Duff, op. cit., p. 70, reports that Herford was taken to Cromwell in October 1539 following publication of an heretical book printed in St Albans without date or name of printer.

[46]	S.T.C. 18794 note.

[47]	Florian Cajori, A History of Mathematics (1893; Chelsea Pubishing Company, New York, 4th edition, 1985), p. 128.

[48]

Despite Joy Easton's bibliographical researches on the editions of Recorde's Groundes, there is still no definitive list of editions (J. Easton, Isis [1967]). S.T.C. gives sixteen editions in the sixteenth century, and eleven for the seventeenth. The edition of 1552 was certainly enlarged by Recorde, and the later 'revised' versions by Dee and Mellis are very little different.

[49]	Duff, op. cit., pp. 171-2.

[50]	For the purpose of this paper, in line with D.N.B. and S.T.C., R. Wolfe is regarded as one person.

[51]	A.Wood, Athenae Oxonienses (2 vols., Oxford, 1691-2), I, col. 84.

[52]	Introduction by R. L. Poole to John Bale, Index Brittaniae Scriptorum... (Oxford, 1902). Bale's original account, Scriptorum Illustrum Brytannie, quam nunc Angliam & Scotiam uocant. Catalogus (Basle, 1557).

[53]

Cajori, History of Mathematics, p. 123. As put by Leonardo of Pisa, '7 old women go to Rome; each woman has 7 mules, each mule carries 7 sacks, each sack contains 7 loaves, with each loaf are 7 knives, each knife is put up in 7 sheaths. What is the sum total of all named. Ans. 137,256.' Reference is made by Cajori to the Egyptian papyrus written by Ahmes some time before 1700 BC, believed to stem from a work of 3400 BC.

[54]

Many of the sources in scientific and mathematical matters dating from around 1200-70 arose from the absorption of Aristotle, Plato, Euclid and Ptolemy into the mainstream of doctrinally acceptable philosophy by the Church authorities. A major problem was that the immediate sources were Arabic and not Christian, and another was that the ancient authors pre-dated Christ.     St Thomas Aquinas attempted to reconcile this dilemma, and one outcome was the promulgation of the Condemnation of 1277, listing 219 prohibited Propositions (mainly contemporary interpretations of Aristotle's philosophical and scientific works). This severely inhibited new research and development of 'approved' ancient sources, under threat of the Inquisition. Although there were a few scholars active during the next two hundred years (Buridan in Paris, Bradwardine in Oxford, for example), very little advance in the physical sciences was made before the printing opened up the field again.:raw-html:     Consequently, many of the early printed works in Latin and in the vernaculars were catching-up, as it were, and their best sources were from the time before the restrictions were introduced. This helps to explain why even in books not even connected to the works of, say, Aristotle, reference was made to his 'approved' authority in order to attempt to allay the suspicions of the extreme elements in the Church hierarchy.

[55]	A fifteenth-century translation into English is reprinted in Robert Steele, The Earliest English Arithmetics (E.E.T.S. Extra Series No. cxviii, 1922 for 1916). Note on printing, p. vi.

[56]

Recorde was well aware of sources as a scholar in the Anglo-Saxon language(s), and his library contained a vast number of medieval scientific works, mainly by scholars of Merton College, Oxford. These are found in Bale's Scriptorum illustrum maioris Brytannie... (1557); reprinted and introduced by R. L. Poole, Index Brittaniae Scriptorum... (Oxford, 1902).

[57]	Taylor, op. cit., p. 5.

[58]

See F. R. Johnson and S. V. Larkey, 'Robert Recorde's Mathematical Teaching and the Anti-Aristotelian Movement', The Huntingdon Library Bulletin, 7 (April 1935). This is not a simple matter, and Popper contributed much of value in distinguishing 'passive' and 'active' traditions from ancient times. Karl Popper, 'Towards a Rational Theory of Tradition', in Conjectures and Refutations (Routledge and Kegan Paul, London, 1963, fifth edition 1974).     The Church may be seen as 'conservative', and the new scientists 'radical' or critical'; both acknowledging the ancients as their fundamental authority. Until Peter Ramus.

[59]	Fellowship was a system, sanctioned by St Thomas Aquinas, of forming partnerships with profit and loss accounts, allowing distribution of shares and avoiding usury in the use of risk capital.

[60]	A comprehensive study is in F. P. Barnard, The Casting-Counter and the Counting Board (Oxford, 1916).

[61]	A current dictionary of mathematics relates that the 'rule of three' is from the nineteenth century.

[62]

Richard Foster Jones, Ancient and Moderns (Washington University Studies. St. Louis, 1961), p. 10, is not alone in misunderstanding the situation in his criticism of mathematical works in the sixteenth century as being 'largely elementary treatises to teach the uninformed'. He is simply unaware of the practical nature of the work being done, and thereby prolongs the battle of those who denigrate the 'merely practical' into the present century. W. W. Rouse Ball, in his History of the Study of Mathematics at Cambridge (Cambridge, 1889) p. 13, remarks that in 1570 fresh statutes were introduced excluding mathematics from undergraduate study 'presumably because this study pertained to practical life, and could, therefore, have no claim to attention in a university.'

[63]	First printed in Venice in 1499, then by Pynson, London, c.1510.

[64]	Recorde was from Pembroke, Dee and Salysburye were of Welsh origin, and of course the Tudors were also Welsh. J. O. Halliwell, Connexion of Wales with the Early Science of England (London, 1840), is remiss in treating only Recorde.

[65]	The word 'pathway' was first used in English by Tyndale (O.E.D.). There can be little doubt that in his mind and in Recorde's were the Latin quadrivium and trivium — also pathways to knowledge. Recorde enjoyed puns, wordplay and conceits.

[66]

Reported in L. Digges, Pantometria (1571), and again by William Bourne, Treasure for Travellers (1578), reprinted in J. O. Halliwell, Rara Mathematica John William Parker, London, 1839). Dee had a large collection of manuscripts by Roger Bacon, in which are described his similar researches of the thirteenth century. It seems most likely that Dee discussed the Bacon manuscripts with L. and T. Digges.

[67]	What has not been understood by a reporter or chronicler has often been ascribed to magic of some sort; sometimes magic as in 'mystery' or 'miracle', other times as in 'witchcraft' or 'conjuring with demons'. It is little different today.

[68]	Here is the almost obligatory key-phrase of the neo-Platonists, from the Book of Wisdom.

[69]	Preface, opening words.

[70]	Dictionary of Scientific Biography (C. C. Gillispie ed., Scribner's, New York, 16 vols., 1970-80), 'Recorde'.

[71]	For Cheke's letter, see pp. 204-6; William Turner, The Names of Herbes in Greke, Latin, Englische, Duche and Frenche wyth the Commune Names that Heraries and Apotecaries Use (Day and Seres, London 1548). Turner was a fellow of Pembroke Hall, Cambridge, and probably knew Cheke.

[72]	F.R. Johnson, Astronomical Thought, seems to have first treated this subject.

[73]	L.Hogben and M. Cartwright, The vocabulary of science (Heinemann, London, 1969), p. 23, provides a schedule.

[74]	F.Cajori, A History of Elementary Mathematics (Macmillan, New York, 1896, revised and enlarged 1917, 1929), p. 137 explains that the 'asses bridge' is the fifth theorem of Euclid.

[75]	The literature on this type of 'square' is now extensive, most recently, R, Bechman, Villard de Honnecourt (Picard, Paris, 1993). Villard de Honnecourt was actively compiling his sketchbooks c.1240.

[76]	King's College Chapel (1446-1515), Magdelene (founded 1542) and Trinity (1546), would have provided sufficient building activity in the medieval manner for the use of that particular square. There was no place for it in Renaissance architecture.

[77]	R.F. Johnson, Astronomical Thought, p. 132. 'No other book of its type, either is Latin or in one of the European vernaculars, rivals it in its scholarship, literary style, and truly scientific attitude toward ancient authorities.'

[78]	Acts of the Privy Council, 1556. Star Chamber, Westminster, xx July 1556. R. Wolfe and (an untraced) John Wykes stood surety for Recorde's bail, which prohibited him leaving the locality in which he lived. 'Therle Pembroke' sitting on the Council.

[79]	The use of this phrase suggests the continuance of an idiom formed in a society not fully literate, when the spoken word was the norm.

[80]	D.N.B. and Frances Margaret Clarke, 'New Light on Robert Recorde', Isis, 8 (1926), p. 55.

[81]	These are too numerous to go into here, but the entry for him in the D.N.B., cross-checked against events and timings in the lives and deaths of Cheke, Digges, and Recorde, is suggestive.

[82]	A topical reference to the first translation into English of Boethius, 1556.

[83]

Antonia McLean, Humanism and the rise of science in Tudor England (Neale Watson A.P. Inc., New York, 1972), p. 19, where a 'technical advance' or a development in an 'attitude of mind' is discussed. Legibility and social acceptability are certainly relevant. Pynson, printer to Henry VII and VIII, brought roman typeface to London in 1509, but it seems only to have been used for works in Latin until this time.

[84]

C.S. Lewis, 'Wit', Studies in Words (Cambridge U.P., 2nd edition, 1967), 'If a man had time to study the history of one word only, wit would perhaps be the best word he could chose.' At Recorde's time the meaning was 'intelligence'. But it was also the subject of a Latin pun from the German, see A. de Morgan, Arithmetical Books from the Invention of Printing to the Present Time (Taylor and Walton, London, 1847), p. 21 (and later versions by David E. Smith, 1915, and Rupert Hall, 1967).

[85]

Recorde's principal new source is Johannes Scheubel (1545, 1551), whom he credits. But also Christoff Rudolf (1525) and Michael Stifel (1544, 1545, 1553). Their work is briefly reviewed in Cajori, A History of Mathematical Notations (The Open Court Publishing Company, La Salle, IL, 1928), I, pp. 133-51.

[86]	'Cossike woorkes' was the German expression for algebra.

[87]	One example of many: Cajori, mathematical notations, pp. 190-99, provides four pages of signs and their uses from the influential works of William Oughtred in the 1630s.

[88]	Ibid., pp. 197, 265-6.

[89]	Taylor, op. cit., p. 313

[90]

Cajori, op. cit., pp. 270-72, 275. In 1923 The Report to the Mathematical Association of America decided to 'make more use of the fractional form and (where the meaning is clear) of the symbol /, and to drop the symbol ÷ in writing algebraic expression.' On the normal computer keyboard the US standard prevails today.

[91]	Ibid., pp. 297-309.

[92]	For the significance of inequality in mathematics, see Rosalind Tanner, 'On the role of equality and inequality in the history of mathematics', The British Journal the History of Science, I, no. 2 (1962-3), pp. 159-89.

[93]

Thomas Har(r)iot (1560-1621); together with William Gilbert (c.1540-1603), two the leading scientists in Europe at the turn of the century; but Hariot published nothing. From his manuscripts his friend Nathaniel Torporley prepared Artis ana lyticae praxis... for publication in 1631; it contained these important signs. For a short review of Hariot see MacLean, op. cit., pp. 150-55. Extract cited in Cajori, Mathematical Notations, pp. 199-200. Also Tanner, op. cit.

[94]	Cajori, Mathematical Notations, p. 168 for Dee; and pp. 285-97 for subsequent developments.

[95]	Ibid., pp. 413-19.

[96]

But it is not true to say that 'With Recorde's addition of the "equal" sign this algebra became completely symbolic' — Gillispie, Dictionary of Scientific Biography, 'Recorde'. The introduction of letters in place of numerals is of significance in the .e~elopment of symbolic algebra; and this is first found in the work of Hariot and Viete in the 1590s.

[97]	O.Wilde, The Happy Prince (1888), reprinted in The Complete Illustrated Stories, Plays and Poems of Oscar Wilde (The Chancellor Press, London, 1986), p. 186.

[98]	Baldassare Castiglione, Il Cortegiano (1528), translated by Hoby as The Book of the Courtier (London, 1561; reprinted J. M. Dent and Sons, London, 1974), Cheke's letter prefaces Hoby's translation.

[99]	D.N.B. gives 1571?, other standard references 1559?

[100]

Wood, op. cit., I, col. 142.

[101]

Edward Fiennes de Clinton, 9th Lord of Clinton and Saye, Earl of Lincoln 1512-85). Appointed lord high admiral 14 May 1550 by his old friend Northumberland, and governor of the Tower in 1553 as a part of the Lady Jane Grey conspiracy. Managed to retain favour of Mary on death of Northumberland, and assisted in quelling Wyatt's rebellion. His relationship to Digges, then, is curious.

[102]

Calendar of the Patent Rolls, 1555-7, p. 44.

[103]

D.N.B. tantalizingly refers to a document concerning him in the fifth year of Elizabeth's reign, 'though it is not printed in the statutes'.

[104]

Taylor, op. cit., pp. 22-3.

[105]

Ibid., p. 165.

[106]

D.Chilton, 'Land Measurement in the Sixteenth Century', Transactions of the Newcomen Society, XXXI (1957-8 and 1958-9), p.124.

[107]

E.G.R. Taylor, Tudor Geography 1485-1583 (Methuen & Co., London, 1930), V-VII.

[108]

End of the section on architecture, reproduced in Frances Yates, Theatre of the World (1969; Routledge and Kegan Paul, London, 1987). John Day (1522-84) was the publisher for the Elements of Euclid, and was the most notable native printer of his generation, publishing also the musical works of Thomas Tallis (as with Greek and arithmetic, discussed with regard to R. Wolf, requiring considerable technical skill), and Foxe's Actes and Monuments (1563).

[109]

E.G.R. Taylor, op. cit., p. 76, lists many of his contacts.

[110]

'Dee's mathematical Preface is of greater importance than Francis Bacon's Advancement of Learning, published thirty-five years later, for Dee fully undestood and emphasized the basic importance of mathematical studies for the advancement of science' — Yates, op. cit., p. 5. Although Debus has produced short introduction to the Preface, work on the Elements itself is lacking, and would prove valuable. The original translation is thought not to have been by Billingsley at all, and there was a nineteenth-century controversy about Dee being the translator. Dee's hand can be seen in the editing, chapter headings and 'annotations and inuentions' at the end of Book X.

[111]

Precedents for such scientific divisions emanate from the lineage of Isidore of Seville, al-Farabi, Hugh of St Victor and Gundisalvo (from the seventh to the twelfth centuries).

[112]

The mystical (Dionysian) side of Dee comes out in his belief that in naming something he somehow invents it.

[113]

Source not only for the 'perspective glass' tests by Dee and Digges, but also one of the very few scientists referred to by Leonardo da Vinci. The contents of Dee's library confirms that the primary source material for the Reformation scientist was medieval, including translations from about the turn of the millennium of Arab works; some original and some in translation from the Greek. Similarly Recorde's library.

[114]

The only recently deceased John Leland (1506?-52) had tried, with limited success, to convince Cromwell in the late 1530s to extend his remit as King's Antiquary to collect on behalf to the king's library beacuse German students ransacking the repositories of the manuscripts.

[116]

John Foxe, in Actes and Monuments (1563), referred to Dee as 'the great Conjurer', and, despite a later retraction, this appellation stuck beyond the grave. By 1570 Dee must have forgiven the printer of both works, John Day.

[117]

Yates, op. cit., p. 17, citing Wood, Athenae Oxonienses.

[118]

Supplication reprinted in Thomas Hearne, Johannis, Confratis & Monachi... (Oxford, 1726), pp. 490-95.

[119]

The bulk of Dee's original work remained in manuscript, and much is now lost.

[120]

This first edition of 1571 is no doubt the source of the opinion that Leonard was alive then, but reading the Preface reveals that he is not; though when he died is not stated.

[121]

A full review of the contents in terms of medieval geometry will have to await another day, for the history of medieval geometry has not yet been written.

[122]

See n. 66. Hariot tells of using 'perspective glasses' in his Report on Ralegh's expedition to Virginia in 1585; see MacLean, op. cit., p. 150.

[123]

Descartes (1596-1650), Discours sur la methode... (Leyden, 1637).

[124]

Gillispie, op. cit., 'Thomas Digges', seems to be alone among the references to have noticed this clue.

[125]

There is as yet no authoritative consideration of the entire work of Thomas Digges. For astronomy, see Johnson, op. cit., and for mathematics, see Taylor, The Mathematical Practitioners.